Huanyao Sun
Diversified Top-k Partial MaxSAT Solving
May 31, 2017
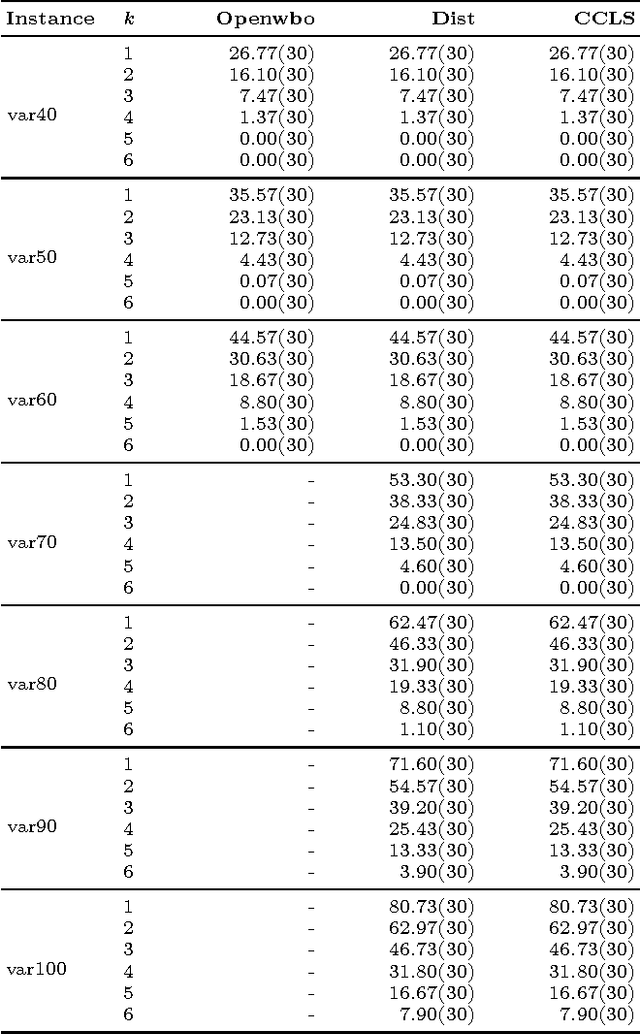
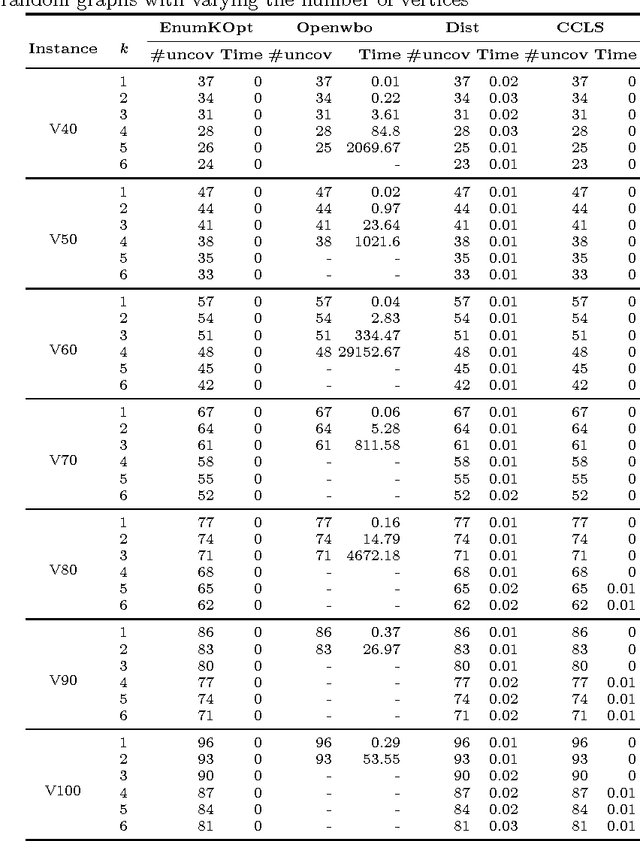
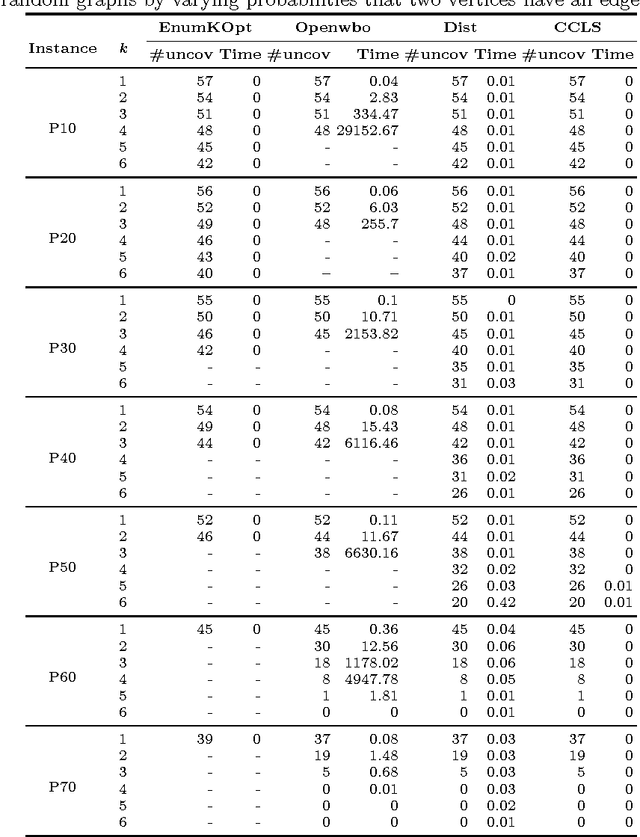
Abstract:We introduce a diversified top-k partial MaxSAT problem, a combination of partial MaxSAT problem and enumeration problem. Given a partial MaxSAT formula F and a positive integer k, the diversified top-k partial MaxSAT is to find k maximal solutions for F such that the k maximal solutions satisfy the maximum number of soft clauses of F. This problem can be widely used in many applications including community detection, sensor place, motif discovery, and combinatorial testing. We prove the problem is NP-hard and propose an approach for solving the problem. The concrete idea of the approach is to design an encoding EE which reduces diversified top-k partial MaxSAT problem into partial MaxSAT problem, and then solve the resulting problem with state-of-art solvers. In addition, we present an algorithm MEMKC exactly solving the diversified top-k partial MaxSAT. Through several experiments we show that our approach can be successfully applied to the interesting problem.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge