Learning While Repositioning in On-Demand Vehicle Sharing Networks

We consider a network inventory problem motivated by one-way, on-demand vehicle sharing services. Due to uncertainties in both demand and returns, as well as a fixed number of rental units across an $n$-location network, the service provider must periodically reposition vehicles to match supply with demand spatially while minimizing costs. The optimal repositioning policy under a general $n$-location network is intractable without knowing the optimal value function. We introduce the best base-stock repositioning policy as a generalization of the classical inventory control policy to $n$ dimensions, and establish its asymptotic optimality in two distinct limiting regimes under general network structures. We present reformulations to efficiently compute this best base-stock policy in an offline setting with pre-collected data. In the online setting, we show that a natural Lipschitz-bandit approach achieves a regret guarantee of $\widetilde{O}(T^{\frac{n}{n+1}})$, which suffers from the exponential dependence on $n$. We illustrate the challenges of learning with censored data in networked systems through a regret lower bound analysis and by demonstrating the suboptimality of alternative algorithmic approaches. Motivated by these challenges, we propose an Online Gradient Repositioning algorithm that relies solely on censored demand. Under a mild cost-structure assumption, we prove that it attains an optimal regret of $O(n^{2.5} \sqrt{T})$, which matches the regret lower bound in $T$ and achieves only polynomial dependence on $n$. The key algorithmic innovation involves proposing surrogate costs to disentangle intertemporal dependencies and leveraging dual solutions to find the gradient of policy change. Numerical experiments demonstrate the effectiveness of our proposed methods.

Smootheness-Adaptive Dynamic Pricing with Nonparametric Demand Learning

We study the dynamic pricing problem where the demand function is nonparametric and H\"older smooth, and we focus on adaptivity to the unknown H\"older smoothness parameter $\beta$ of the demand function. Traditionally the optimal dynamic pricing algorithm heavily relies on the knowledge of $\beta$ to achieve a minimax optimal regret of $\widetilde{O}(T^{\frac{\beta+1}{2\beta+1}})$. However, we highlight the challenge of adaptivity in this dynamic pricing problem by proving that no pricing policy can adaptively achieve this minimax optimal regret without knowledge of $\beta$. Motivated by the impossibility result, we propose a self-similarity condition to enable adaptivity. Importantly, we show that the self-similarity condition does not compromise the problem's inherent complexity since it preserves the regret lower bound $\Omega(T^{\frac{\beta+1}{2\beta+1}})$. Furthermore, we develop a smoothness-adaptive dynamic pricing algorithm and theoretically prove that the algorithm achieves this minimax optimal regret bound without the prior knowledge $\beta$.

Potential Energy Advantage of Quantum Economy

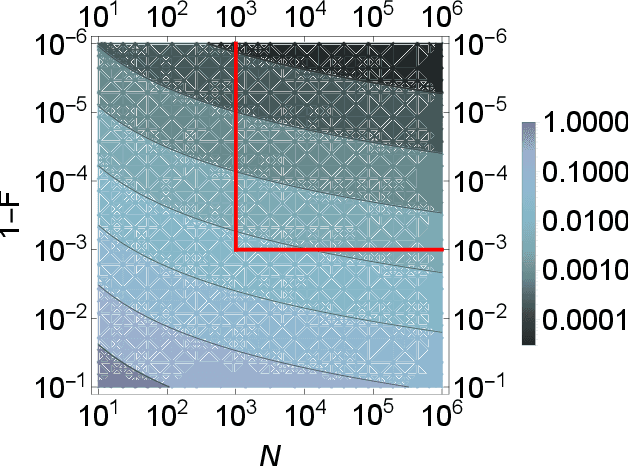
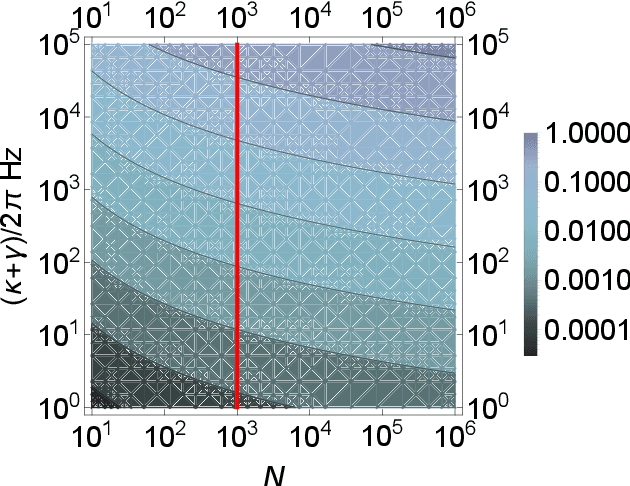
Energy cost is increasingly crucial in the modern computing industry with the wide deployment of large-scale machine learning models and language models. For the firms that provide computing services, low energy consumption is important both from the perspective of their own market growth and the government's regulations. In this paper, we study the energy benefits of quantum computing vis-a-vis classical computing. Deviating from the conventional notion of quantum advantage based solely on computational complexity, we redefine advantage in an energy efficiency context. Through a Cournot competition model constrained by energy usage, we demonstrate quantum computing firms can outperform classical counterparts in both profitability and energy efficiency at Nash equilibrium. Therefore quantum computing may represent a more sustainable pathway for the computing industry. Moreover, we discover that the energy benefits of quantum computing economies are contingent on large-scale computation. Based on real physical parameters, we further illustrate the scale of operation necessary for realizing this energy efficiency advantage.

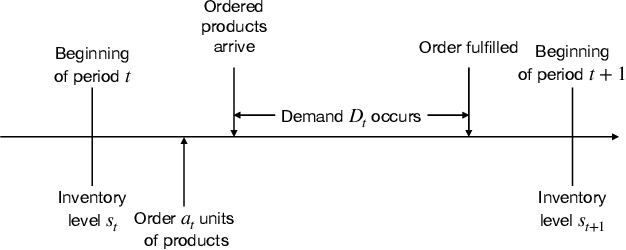
Quantum Computing Methods for Supply Chain Management

Quantum computing is expected to have transformative influences on many domains, but its practical deployments on industry problems are underexplored. We focus on applying quantum computing to operations management problems in industry, and in particular, supply chain management. Many problems in supply chain management involve large state and action spaces and pose computational challenges on classic computers. We develop a quantized policy iteration algorithm to solve an inventory control problem and demonstrative its effectiveness. We also discuss in-depth the hardware requirements and potential challenges on implementing this quantum algorithm in the near term. Our simulations and experiments are powered by the IBM Qiskit and the qBraid system.