DeepOPF-U: A Unified Deep Neural Network to Solve AC Optimal Power Flow in Multiple Networks

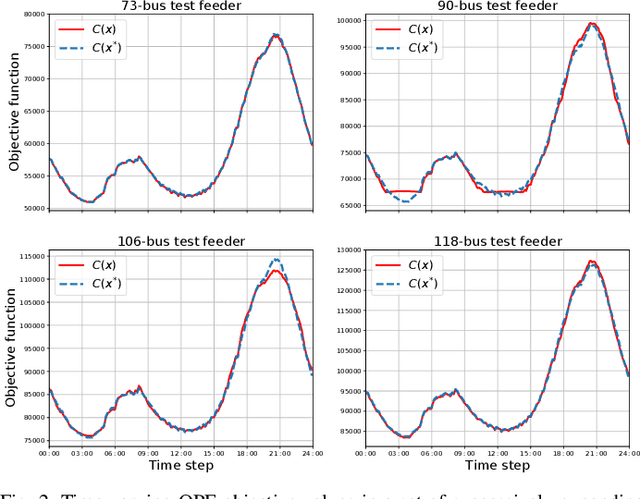
The traditional machine learning models to solve optimal power flow (OPF) are mostly trained for a given power network and lack generalizability to today's power networks with varying topologies and growing plug-and-play distributed energy resources (DERs). In this paper, we propose DeepOPF-U, which uses one unified deep neural network (DNN) to solve alternating-current (AC) OPF problems in different power networks, including a set of power networks that is successively expanding. Specifically, we design elastic input and output layers for the vectors of given loads and OPF solutions with varying lengths in different networks. The proposed method, using a single unified DNN, can deal with different and growing numbers of buses, lines, loads, and DERs. Simulations of IEEE 57/118/300-bus test systems and a network growing from 73 to 118 buses verify the improved performance of DeepOPF-U compared to existing DNN-based solution methods.

Differentially Private Stochastic Convex Optimization in (Non)-Euclidean Space Revisited

In this paper, we revisit the problem of Differentially Private Stochastic Convex Optimization (DP-SCO) in Euclidean and general $\ell_p^d$ spaces. Specifically, we focus on three settings that are still far from well understood: (1) DP-SCO over a constrained and bounded (convex) set in Euclidean space; (2) unconstrained DP-SCO in $\ell_p^d$ space; (3) DP-SCO with heavy-tailed data over a constrained and bounded set in $\ell_p^d$ space. For problem (1), for both convex and strongly convex loss functions, we propose methods whose outputs could achieve (expected) excess population risks that are only dependent on the Gaussian width of the constraint set rather than the dimension of the space. Moreover, we also show the bound for strongly convex functions is optimal up to a logarithmic factor. For problems (2) and (3), we propose several novel algorithms and provide the first theoretical results for both cases when $1<p<2$ and $2\leq p\leq \infty$.