Diffractive optical system design by cascaded propagation

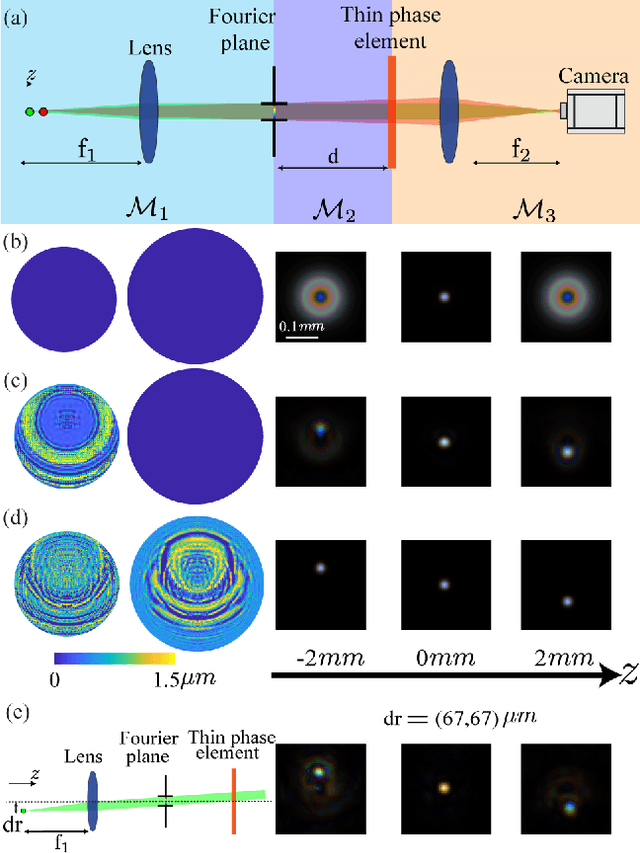
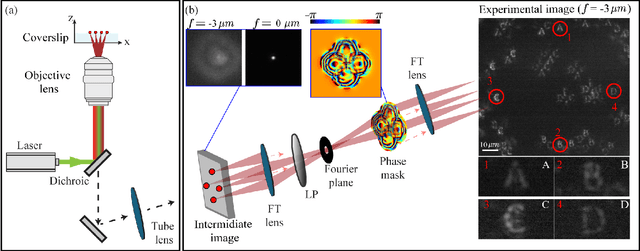
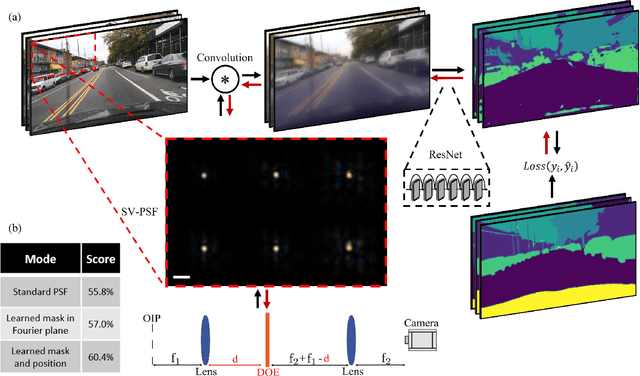
Modern design of complex optical systems relies heavily on computational tools. These typically utilize geometrical optics as well as Fourier optics, which enables the use of diffractive elements to manipulate light with features on the scale of a wavelength. Fourier optics is typically used for designing thin elements, placed in the system's aperture, generating a shift-invariant Point Spread Function (PSF). A major bottleneck in applying Fourier Optics in many cases of interest, e.g. when dealing with multiple, or out-of-aperture elements, comes from numerical complexity. In this work, we propose and implement an efficient and differentiable propagation model based on the Collins integral, which enables the optimization of diffraction optical systems with unprecedented design freedom using backpropagation. We demonstrate the applicability of our method, numerically and experimentally, by engineering shift-variant PSFs via thin plate elements placed in arbitrary planes inside complex imaging systems, performing cascaded optimization of multiple planes, and designing optimal machine-vision systems by deep learning.

Learning an optimal PSF-pair for ultra-dense 3D localization microscopy

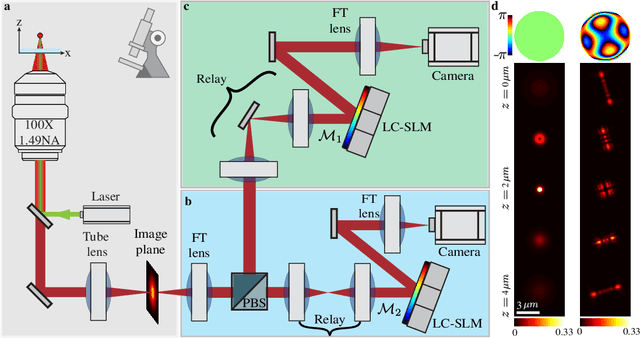
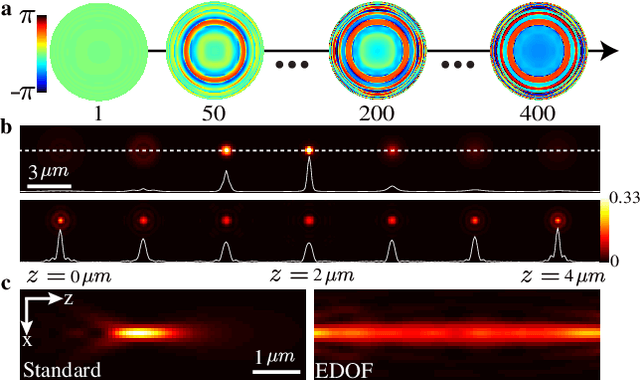
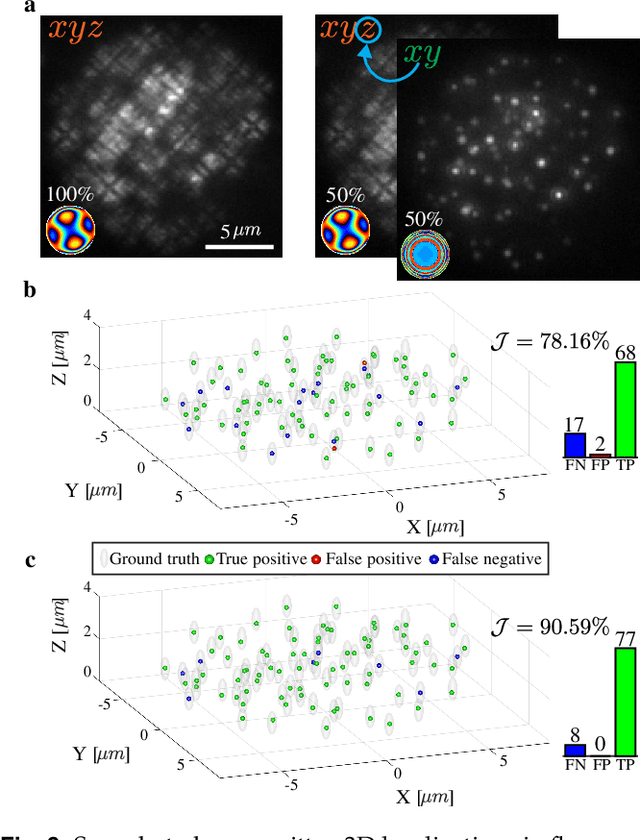
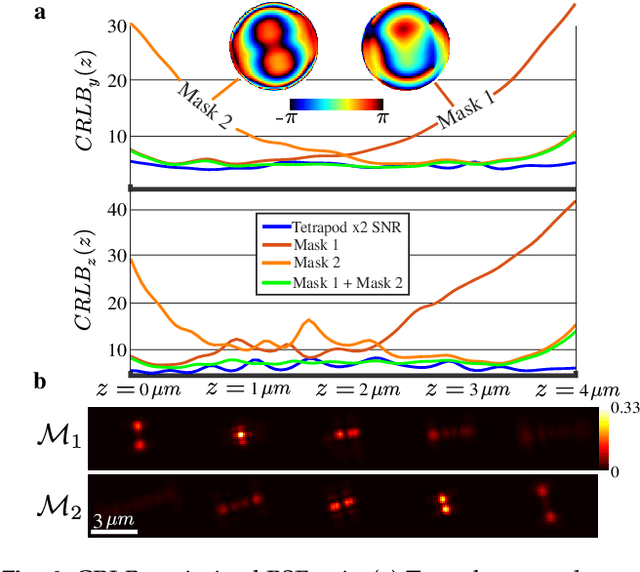
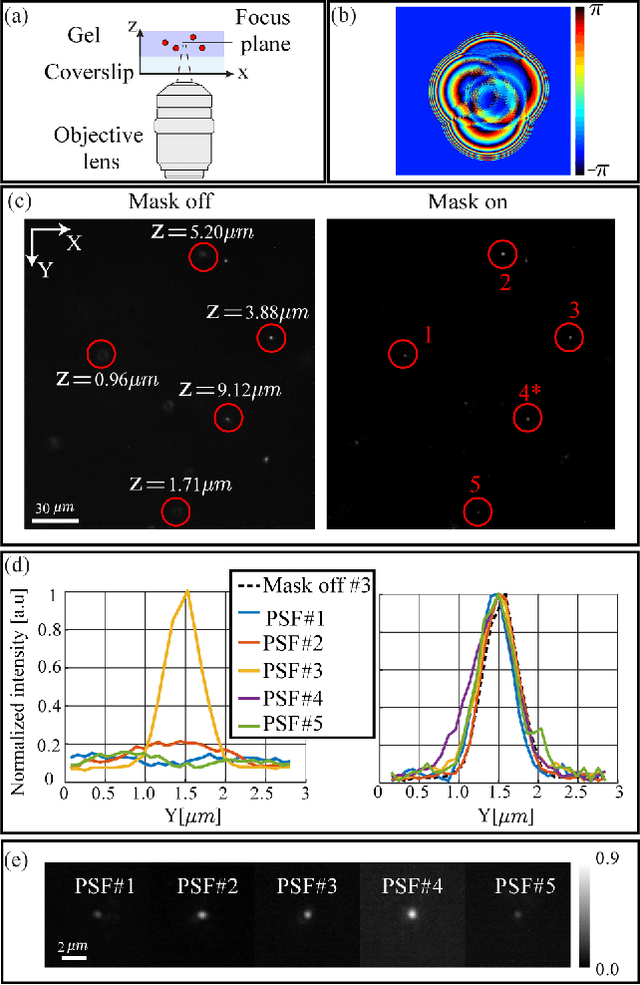
A long-standing challenge in multiple-particle-tracking is the accurate and precise 3D localization of individual particles at close proximity. One established approach for snapshot 3D imaging is point-spread-function (PSF) engineering, in which the PSF is modified to encode the axial information. However, engineered PSFs are challenging to localize at high densities due to lateral PSF overlaps. Here we suggest using multiple PSFs simultaneously to help overcome this challenge, and investigate the problem of engineering multiple PSFs for dense 3D localization. We implement our approach using a bifurcated optical system that modifies two separate PSFs, and design the PSFs using three different approaches including end-to-end learning. We demonstrate our approach experimentally by volumetric imaging of fluorescently labelled telomeres in cells.