Tensor Ring Optimized Quantum-Enhanced Tensor Neural Networks

Quantum machine learning researchers often rely on incorporating Tensor Networks (TN) into Deep Neural Networks (DNN) and variational optimization. However, the standard optimization techniques used for training the contracted trainable weights of each model layer suffer from the correlations and entanglement structure between the model parameters on classical implementations. To address this issue, a multi-layer design of a Tensor Ring optimized variational Quantum learning classifier (Quan-TR) comprising cascading entangling gates replacing the fully connected (dense) layers of a TN is proposed, and it is referred to as Tensor Ring optimized Quantum-enhanced tensor neural Networks (TR-QNet). TR-QNet parameters are optimized through the stochastic gradient descent algorithm on qubit measurements. The proposed TR-QNet is assessed on three distinct datasets, namely Iris, MNIST, and CIFAR-10, to demonstrate the enhanced precision achieved for binary classification. On quantum simulations, the proposed TR-QNet achieves promising accuracy of $94.5\%$, $86.16\%$, and $83.54\%$ on the Iris, MNIST, and CIFAR-10 datasets, respectively. Benchmark studies have been conducted on state-of-the-art quantum and classical implementations of TN models to show the efficacy of the proposed TR-QNet. Moreover, the scalability of TR-QNet highlights its potential for exhibiting in deep learning applications on a large scale. The PyTorch implementation of TR-QNet is available on Github:https://github.com/konar1987/TR-QNet/

Qutrit-inspired Fully Self-supervised Shallow Quantum Learning Network for Brain Tumor Segmentation

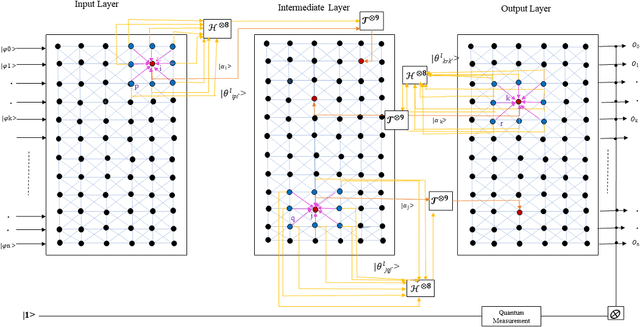
Classical self-supervised networks suffer from convergence problems and reduced segmentation accuracy due to forceful termination. Qubits or bi-level quantum bits often describe quantum neural network models. In this article, a novel self-supervised shallow learning network model exploiting the sophisticated three-level qutrit-inspired quantum information system referred to as Quantum Fully Self-Supervised Neural Network (QFS-Net) is presented for automated segmentation of brain MR images. The QFS-Net model comprises a trinity of a layered structure of qutrits inter-connected through parametric Hadamard gates using an 8-connected second-order neighborhood-based topology. The non-linear transformation of the qutrit states allows the underlying quantum neural network model to encode the quantum states, thereby enabling a faster self-organized counter-propagation of these states between the layers without supervision. The suggested QFS-Net model is tailored and extensively validated on Cancer Imaging Archive (TCIA) data set collected from Nature repository and also compared with state of the art supervised (U-Net and URes-Net architectures) and the self-supervised QIS-Net model. Results shed promising segmented outcome in detecting tumors in terms of dice similarity and accuracy with minimum human intervention and computational resources.