Dataset of polarimetric images of mechanically generated water surface waves coupled with surface elevation records by wave gauges linear array

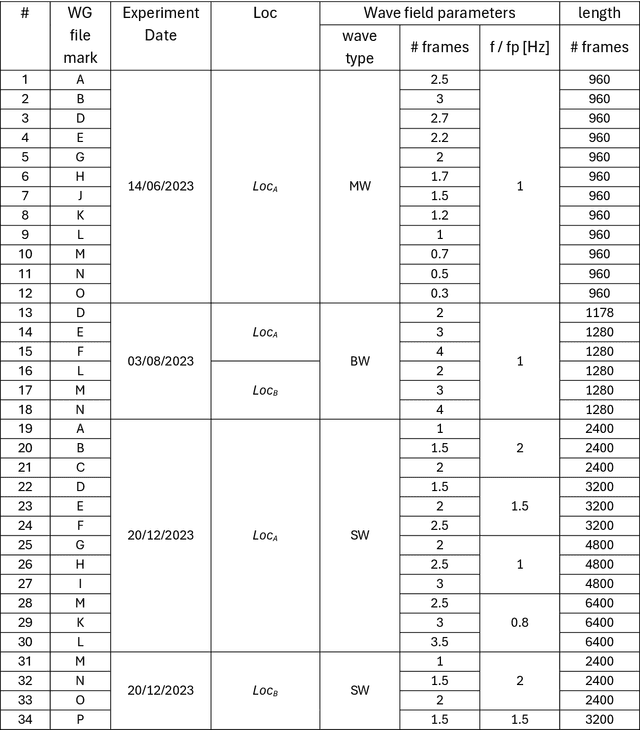
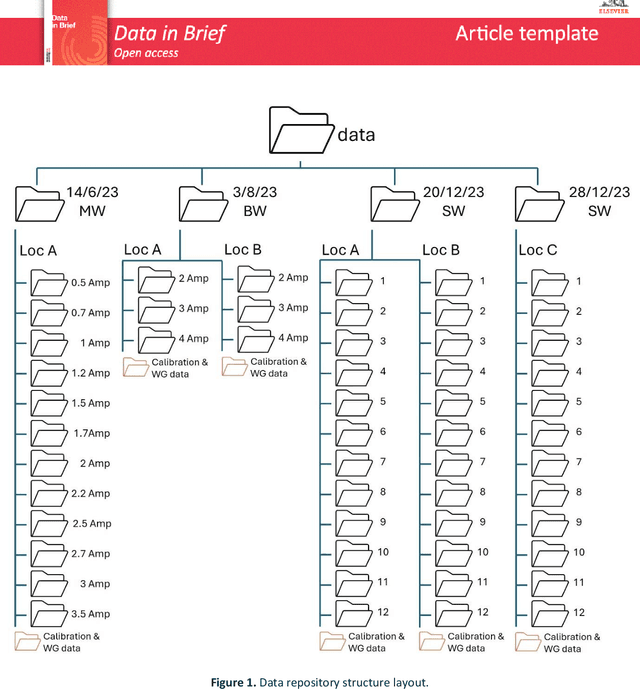
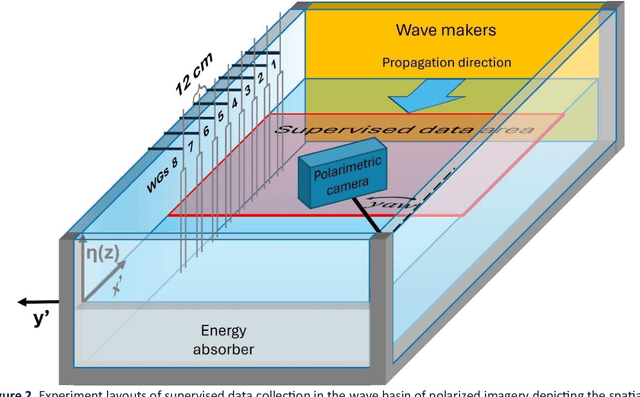
Effective spatio-temporal measurements of water surface elevation (water waves) in laboratory experiments are essential for scientific and engineering research. Existing techniques are often cumbersome, computationally heavy and generally suffer from limited wavenumber/frequency response. To address these challenges a novel method was developed, using polarization filter equipped camera as the main sensor and Machine Learning (ML) algorithms for data processing [1,2]. The developed method training and evaluation was based on in-house made supervised dataset. Here we present this supervised dataset of polarimetric images of the water surface coupled with the water surface elevation measurements made by a linear array of resistance-type wave gauges (WG). The water waves were mechanically generated in a laboratory waves basin, and the polarimetric images were captured under an artificial light source. Meticulous camera and WGs calibration and instruments synchronization supported high spatio-temporal resolution. The data set covers several wavefield conditions, from simple monochromatic wave trains of various steepness, to irregular wavefield of JONSWAP prescribed spectral shape and several wave breaking scenarios. The dataset contains measurements repeated in several camera positions relative to the wave field propagation direction.

Wave (from) Polarized Light Learning (WPLL) method: high resolution spatio-temporal measurements of water surface waves in laboratory setups

Effective spatio-temporal measurements of water surface elevation (water waves) in laboratory experiments are crucial for scientific and engineering research. Existing techniques are often cumbersome, computationally heavy and generally suffer from limitations in wavenumber/frequency response. To address these challenges, we propose Wave (from) Polarized Light Learning (WPLL), a learning based remote sensing method for laboratory implementation, capable of inferring surface elevation and slope maps in high resolution. The method uses the polarization properties of light reflected from the water surface. The WPLL uses a deep neural network (DNN) model that approximates the water surface slopes from the polarized light intensities. Once trained on simple monochromatic wave trains, the WPLL is capable of producing high-resolution and accurate 2D reconstruction of the water surface slopes and elevation in a variety of irregular wave fields. The method's robustness is demonstrated by showcasing its high wavenumber/frequency response, its ability to reconstruct wave fields propagating at arbitrary angles relative to the camera optical axis, and its computational efficiency. This developed methodology is an accurate and cost-effective near-real time remote sensing tool for laboratory water surface waves measurements, setting the path for upscaling to open sea application for research, monitoring, and short-time forecasting.

Identifiable Solutions to Foreground Signature Extraction from Hyperspectral Images in an Intimate Mixing Scenario

The problem of foreground material signature extraction in an intimate (nonlinear) mixing setting is considered. It is possible for a foreground material signature to appear in combination with multiple background material signatures. We explore a framework for foreground material signature extraction based on a patch model that accounts for such background variation. We identify data conditions under which a foreground material signature can be extracted up to scaling and elementwise-inverse variations. We present algorithms based on volume minimization and endpoint member identification to recover foreground material signatures under these conditions. Numerical experiments on real and synthetic data illustrate the efficacy of the proposed algorithms.

Competitive Analysis of Minimum-Cut Maximum Flow Algorithms in Vision Problems

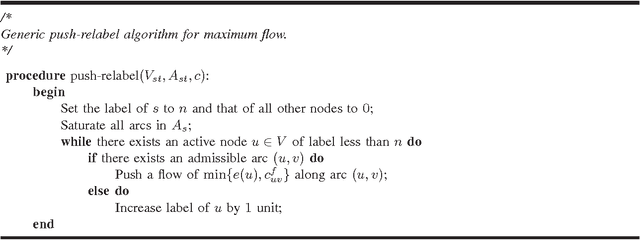
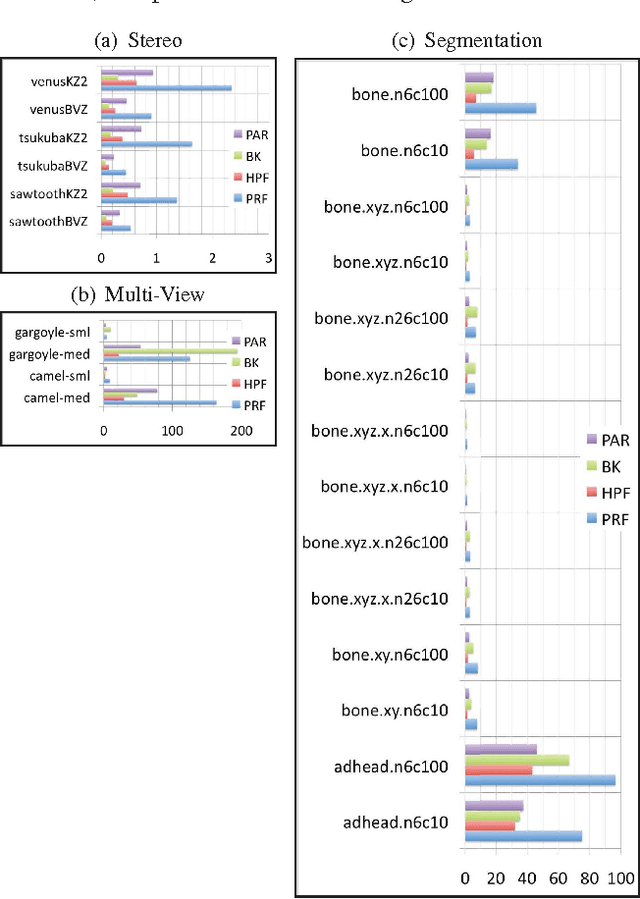
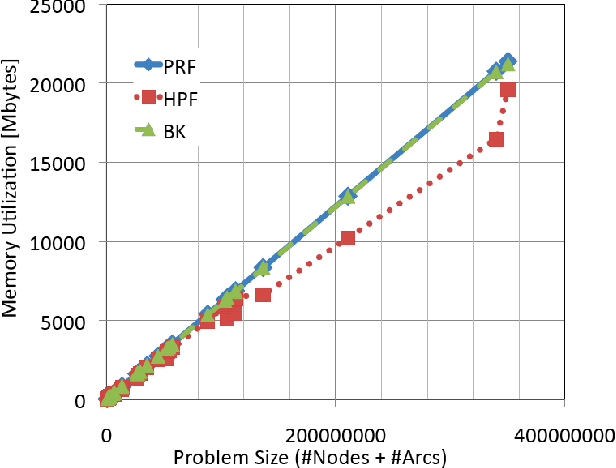
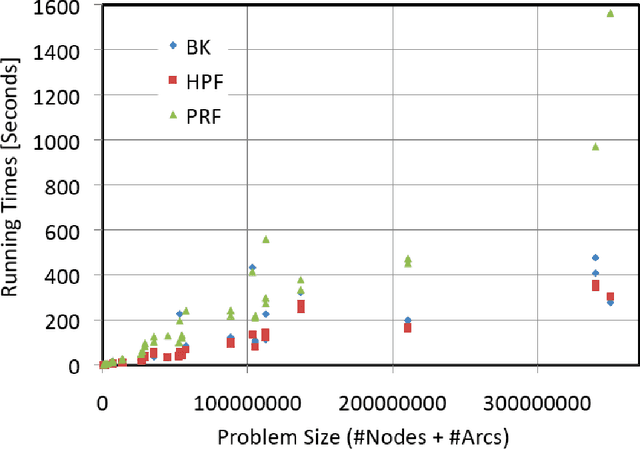
Rapid advances in image acquisition and storage technology underline the need for algorithms that are capable of solving large scale image processing and computer-vision problems. The minimum cut problem plays an important role in processing many of these imaging problems such as, image and video segmentation, stereo vision, multi-view reconstruction and surface fitting. While several min-cut/max-flow algorithms can be found in the literature, their performance in practice has been studied primarily outside the scope of computer vision. We present here the results of a comprehensive computational study, in terms of execution times and memory utilization, of four recently published algorithms, which optimally solve the {\em s-t} cut and maximum flow problems: (i) Goldberg's and Tarjan's {\em Push-Relabel}; (ii) Hochbaum's {\em pseudoflow}; (iii) Boykov's and Kolmogorov's {\em augmenting paths}; and (iv) Goldberg's {\em partial augment-relabel}. Our results demonstrate that the {\em Hochbaum's pseudoflow} algorithm, is faster and utilizes less memory than the other algorithms on all problem instances investigated.