Projected Regression Methods for Inverting Fredholm Integrals: Formalism and Application to Analytical Continuation

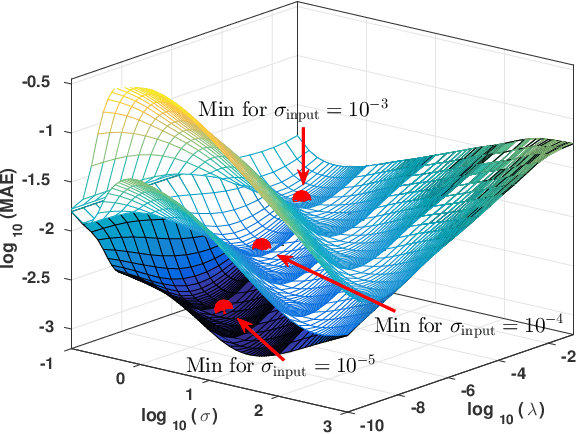
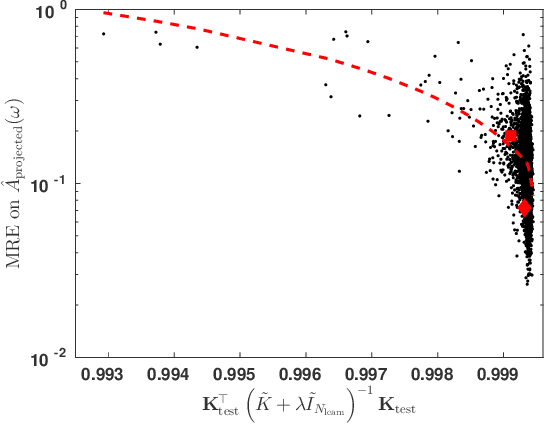
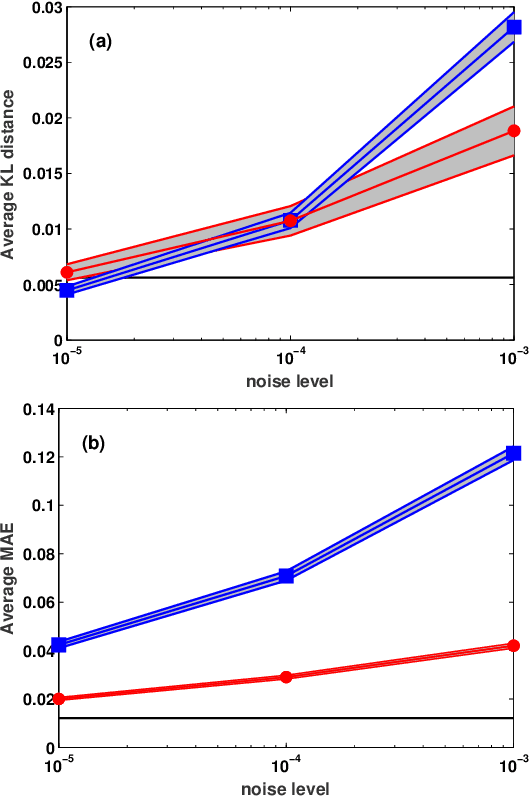
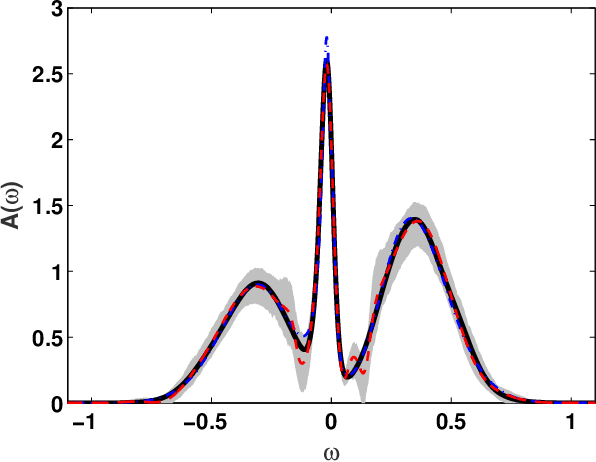
We present a machine learning approach to the inversion of Fredholm integrals of the first kind. The approach provides a natural regularization in cases where the inverse of the Fredholm kernel is ill-conditioned. It also provides an efficient and stable treatment of constraints. The key observation is that the stability of the forward problem permits the construction of a large database of outputs for physically meaningful inputs. We apply machine learning to this database to generate a regression function of controlled complexity, which returns approximate solutions for previously unseen inputs; the approximate solutions are then projected onto the subspace of functions satisfying relevant constraints. We also derive and present uncertainty estimates. We illustrate the approach by applying it to the analytical continuation problem of quantum many-body physics, which involves reconstructing the frequency dependence of physical excitation spectra from data obtained at specific points in the complex frequency plane. Under standard error metrics the method performs as well or better than the Maximum Entropy method for low input noise and is substantially more robust to increased input noise. We expect the methodology to be similarly effective for any problem involving a formally ill-conditioned inversion, provided that the forward problem can be efficiently solved.

Machine learning for many-body physics: efficient solution of dynamical mean-field theory

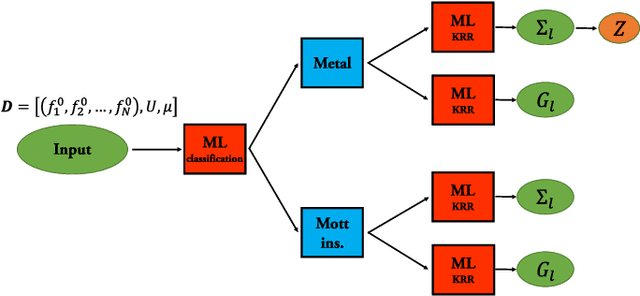
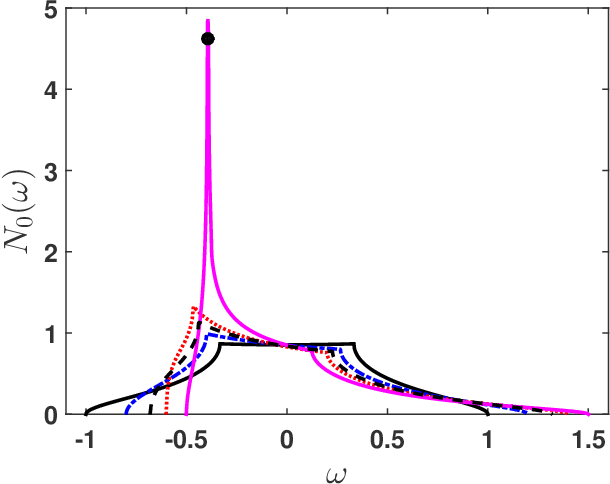
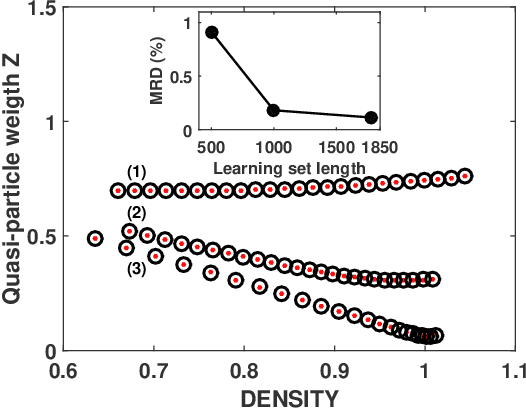
Machine learning methods for solving the equations of dynamical mean-field theory are developed. The method is demonstrated on the three dimensional Hubbard model. The key technical issues are defining a mapping of an input function to an output function, and distinguishing metallic from insulating solutions. Both metallic and Mott insulator solutions can be predicted. The validity of the machine learning scheme is assessed by comparing predictions of full correlation functions, of quasi-particle weight and particle density to values directly computed. The results indicate that with modest further development, machine learning approach may be an attractive computational efficient option for real materials predictions for strongly correlated systems.

Machine learning for many-body physics: The case of the Anderson impurity model

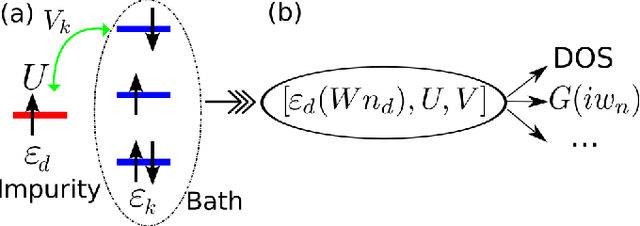
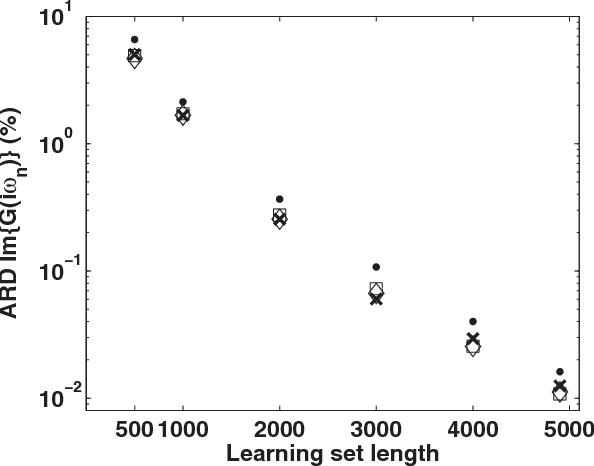
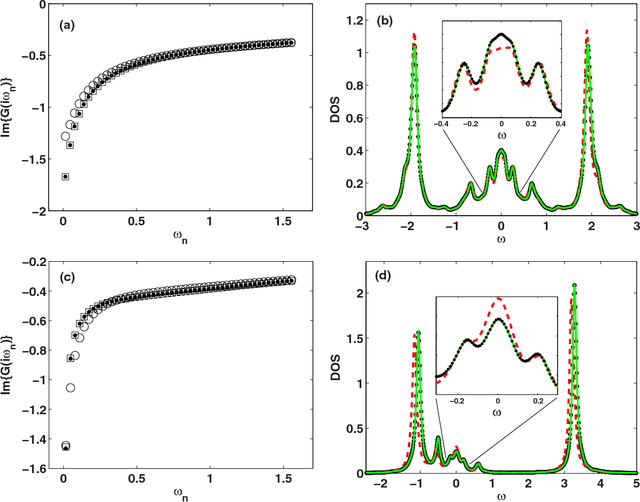
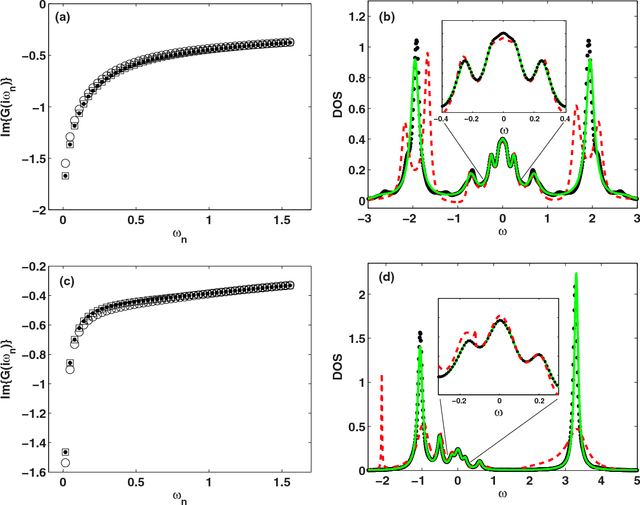
Machine learning methods are applied to finding the Green's function of the Anderson impurity model, a basic model system of quantum many-body condensed-matter physics. Different methods of parametrizing the Green's function are investigated; a representation in terms of Legendre polynomials is found to be superior due to its limited number of coefficients and its applicability to state of the art methods of solution. The dependence of the errors on the size of the training set is determined. The results indicate that a machine learning approach to dynamical mean-field theory may be feasible.