Uncertainty Quantification with Generative Models
Paper and Code
Oct 22, 2019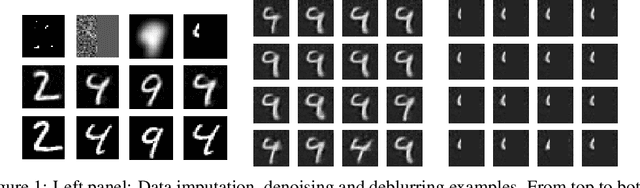

We develop a generative model-based approach to Bayesian inverse problems, such as image reconstruction from noisy and incomplete images. Our framework addresses two common challenges of Bayesian reconstructions: 1) It makes use of complex, data-driven priors that comprise all available information about the uncorrupted data distribution. 2) It enables computationally tractable uncertainty quantification in the form of posterior analysis in latent and data space. The method is very efficient in that the generative model only has to be trained once on an uncorrupted data set, after that, the procedure can be used for arbitrary corruption types.
* accepted submission to the Bayesian Deep Learning NeurIPS 2019
Workshop
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge