Turning machines

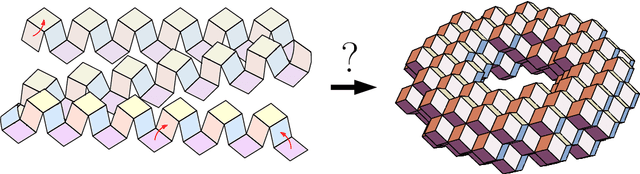
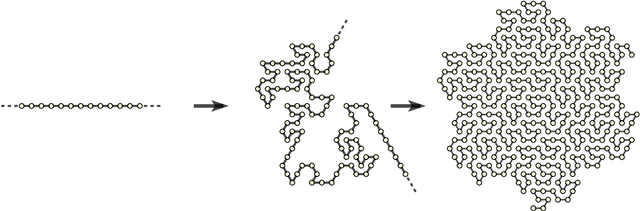
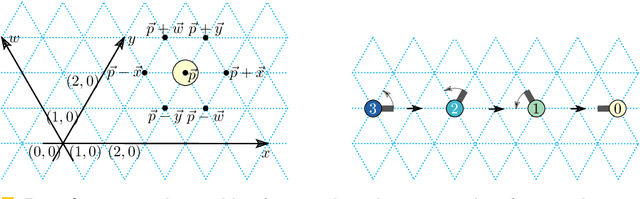
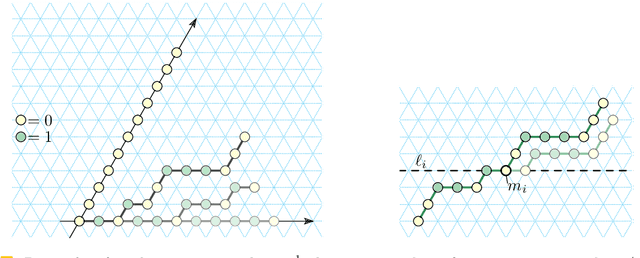
Molecular robotics is challenging, so it seems best to keep it simple. We consider an abstract molecular robotics model based on simple folding instructions that execute asynchronously. Turning Machines are a simple 1D to 2D folding model, also easily generalisable to 2D to 3D folding. A Turning Machine starts out as a line of connected monomers in the discrete plane, each with an associated turning number. A monomer turns relative to its neighbours, executing a unit-distance translation that drags other monomers along with it, and through collective motion the initial set of monomers eventually folds into a programmed shape. We fully characterise the ability of Turning Machines to execute line rotations, and to do so efficiently: computing an almost-full line rotation of $5\pi/3$ radians is possible, yet a full $2\pi$ rotation is impossible. We show that such line-rotations represent a fundamental primitive in the model, by using them to efficiently and asynchronously fold arbitrarily large zig-zag-rastered squares and $y$-monotone shapes.