Top-$K$ Ranking from Pairwise Comparisons: When Spectral Ranking is Optimal
Paper and Code
Mar 14, 2016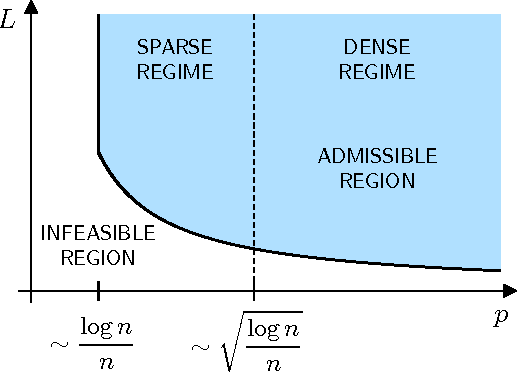
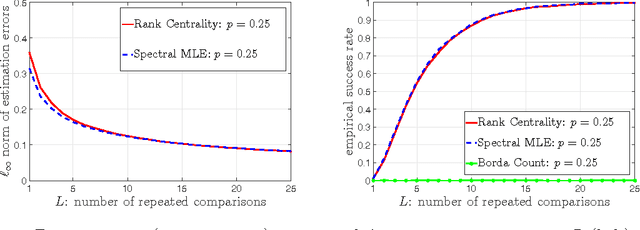
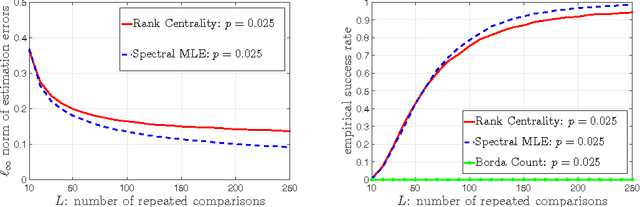
We explore the top-$K$ rank aggregation problem. Suppose a collection of items is compared in pairs repeatedly, and we aim to recover a consistent ordering that focuses on the top-$K$ ranked items based on partially revealed preference information. We investigate the Bradley-Terry-Luce model in which one ranks items according to their perceived utilities modeled as noisy observations of their underlying true utilities. Our main contributions are two-fold. First, in a general comparison model where item pairs to compare are given a priori, we attain an upper and lower bound on the sample size for reliable recovery of the top-$K$ ranked items. Second, more importantly, extending the result to a random comparison model where item pairs to compare are chosen independently with some probability, we show that in slightly restricted regimes, the gap between the derived bounds reduces to a constant factor, hence reveals that a spectral method can achieve the minimax optimality on the (order-wise) sample size required for top-$K$ ranking. That is to say, we demonstrate a spectral method alone to be sufficient to achieve the optimality and advantageous in terms of computational complexity, as it does not require an additional stage of maximum likelihood estimation that a state-of-the-art scheme employs to achieve the optimality. We corroborate our main results by numerical experiments.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge