The Planted Matching Problem: Phase Transitions and Exact Results
Paper and Code
Dec 18, 2019
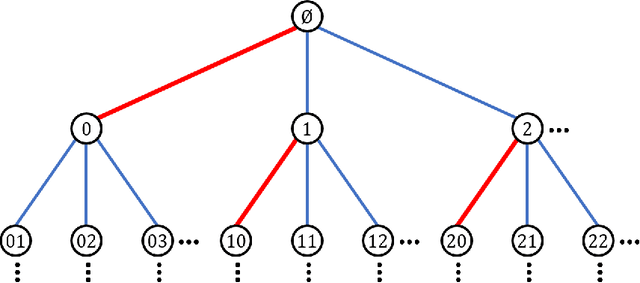
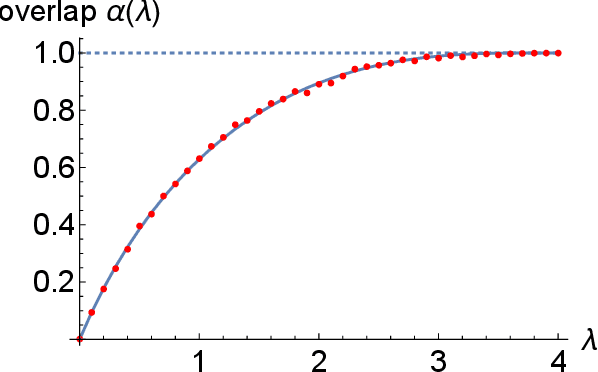
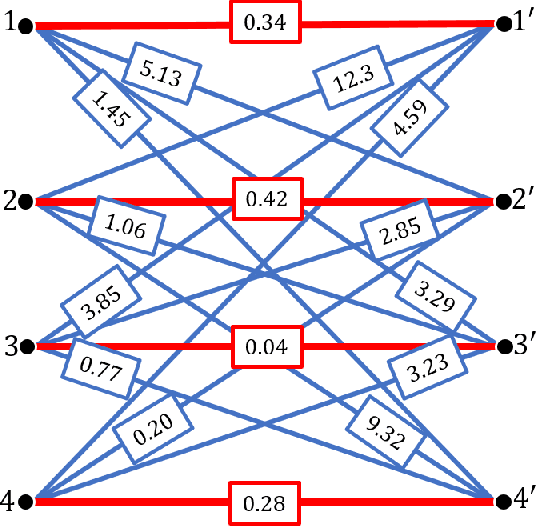
We study the problem of recovering a planted matching in randomly weighted complete bipartite graphs $K_{n,n}$. For some unknown perfect matching $M^*$, the weight of an edge is drawn from one distribution $P$ if $e \in M^*$ and another distribution $Q$ if $e \in M^*$. Our goal is to infer $M^*$, exactly or approximately, from the edge weights. In this paper we take $P=\exp(\lambda)$ and $Q=\exp(1/n)$, in which case the maximum-likelihood estimator of $M^*$ is the minimum-weight matching $M_{\min}$. We obtain precise results on the overlap between $M^*$ and $M_{\min}$, i.e., the fraction of edges they have in common. For $\lambda \ge 4$ we have almost-perfect recovery, with overlap $1-o(1)$ with high probability. For $\lambda < 4$ the expected overlap is an explicit function $\alpha(\lambda) < 1$: we compute it by generalizing Aldous' celebrated proof of M\'ezard and Parisi's $\zeta(2)$ conjecture for the un-planted model, using local weak convergence to relate $K_{n,n}$ to a type of weighted infinite tree, and then deriving a system of differential equations from a message-passing algorithm on this tree.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge