Sampling-Free Variational Inference of Bayesian Neural Nets

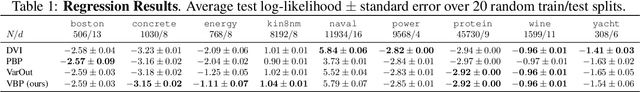
We propose a new Bayesian Neural Net (BNN) formulation that affords variational inference for which the evidence lower bound (ELBO) is analytically tractable subject to a tight approximation. We achieve this tractability by decomposing ReLU nonlinearities into an identity function and a Kronecker delta function. We demonstrate formally that assigning the outputs of these functions to separate latent variables allows representing the neural network likelihood as the composition of a chain of linear operations. Performing variational inference on this construction enables closed-form computation of the evidence lower bound. It can thus be maximized without requiring Monte Carlo sampling to approximate the problematic expected log-likelihood term. The resultant formulation boils down to stochastic gradient descent, where the gradients are not distorted by any factor besides minibatch selection. This amends a long-standing disadvantage of BNNs relative to deterministic nets. Experiments on four benchmark data sets show that the cleaner gradients provided by our construction yield a steeper learning curve, achieving higher prediction accuracies for a fixed epoch budget.