Risk Aware Ranking for Top-$k$ Recommendations
Paper and Code
Apr 12, 2019
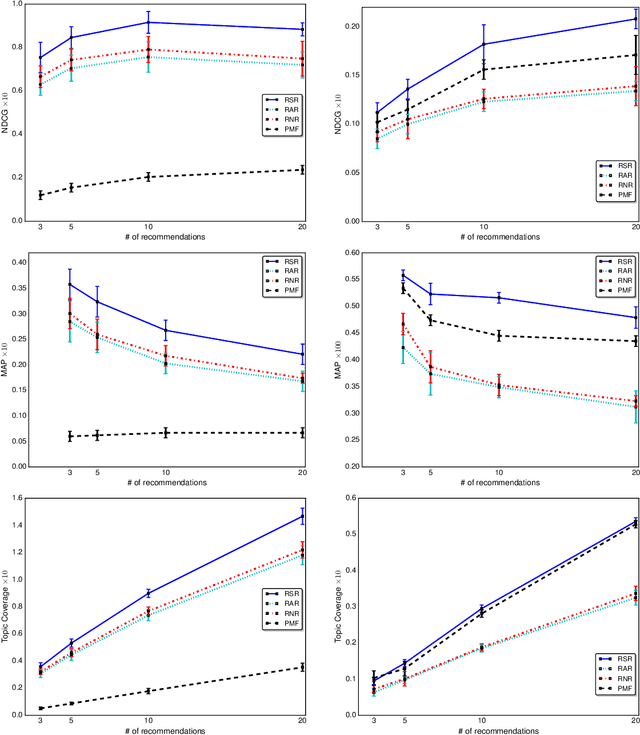
Given an incomplete ratings data over a set of users and items, the preference completion problem aims to estimate a personalized total preference order over a subset of the items. In practical settings, a ranked list of top-$k$ items from the estimated preference order is recommended to the end user in the decreasing order of preference for final consumption. We analyze this model and observe that such a ranking model results in suboptimal performance when the payoff associated with the recommended items is different. We propose a novel and very efficient algorithm for the preference ranking considering the uncertainty regarding the payoffs of the items. Once the preference scores for the users are obtained using any preference learning algorithm, we show that ranking the items using a risk seeking utility function results in the best ranking performance.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge