Real-Time Risk-Bounded Tube-Based Trajectory Safety Verification
Paper and Code
Oct 01, 2021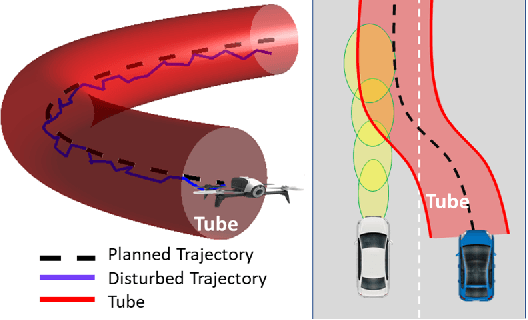

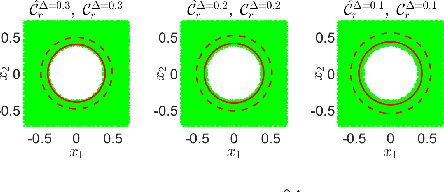

In this paper, we address the real-time risk-bounded safety verification problem of continuous-time state trajectories of autonomous systems in the presence of uncertain time-varying nonlinear safety constraints. Risk is defined as the probability of not satisfying the uncertain safety constraints. Existing approaches to address the safety verification problems under uncertainties either are limited to particular classes of uncertainties and safety constraints, e.g., Gaussian uncertainties and linear constraints, or rely on sampling based methods. In this paper, we provide a fast convex algorithm to efficiently evaluate the probabilistic nonlinear safety constraints in the presence of arbitrary probability distributions and long planning horizons in real-time, without the need for uncertainty samples and time discretization. The provided approach verifies the safety of the given state trajectory and its neighborhood (tube) to account for the execution uncertainties and risk. In the provided approach, we first use the moments of the probability distributions of the uncertainties to transform the probabilistic safety constraints into a set of deterministic safety constraints. We then use convex methods based on sum-of-squares polynomials to verify the obtained deterministic safety constraints over the entire planning time horizon without time discretization. To illustrate the performance of the proposed method, we apply the provided method to the safety verification problem of self-driving vehicles and autonomous aerial vehicles.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge