Principal Trade-off Analysis
Paper and Code
Jun 09, 2022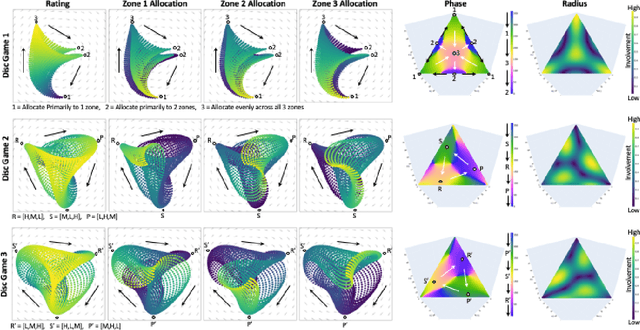
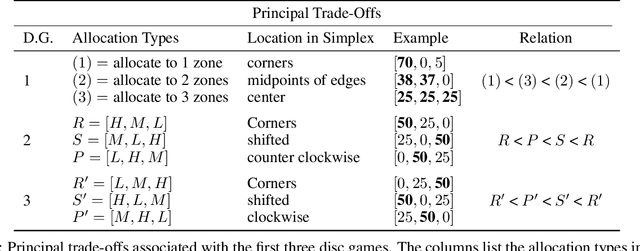
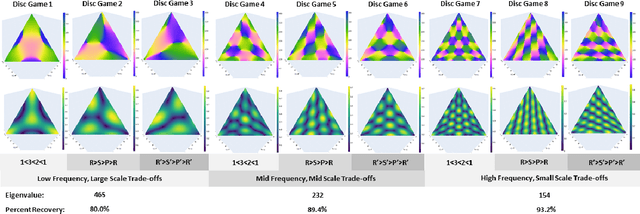

This paper develops Principal Trade-off Analysis (PTA), a decomposition method, analogous to Principal Component Analysis (PCA), which permits the representation of any game as the weighted sum of disc games (continuous R-P-S games). Applying PTA to empirically generated tournament graphs produces a sequence of embeddings into orthogonal 2D feature planes representing independent strategic trade-offs. Each trade-off generates a mode of cyclic competition. Like PCA, PTA provides optimal low rank estimates of the tournament graphs that can be truncated for approximation. The complexity of cyclic competition can be quantified by computing the number of significant cyclic modes. We illustrate the PTA via application to a pair of games (Blotto, Pokemon). The resulting 2D disc game representations are shown to be well suited for visualization and are easily interpretable. In Blotto, PTA identifies game symmetries, and specifies strategic trade-offs associated with distinct win conditions. For Pokemon, PTA embeddings produce clusters in the embedding space that naturally correspond to Pokemon types, a design in the game that produces cyclic trade offs.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge