PCD2Vec: A Poisson Correction Distance-Based Approach for Viral Host Classification
Paper and Code
Apr 13, 2023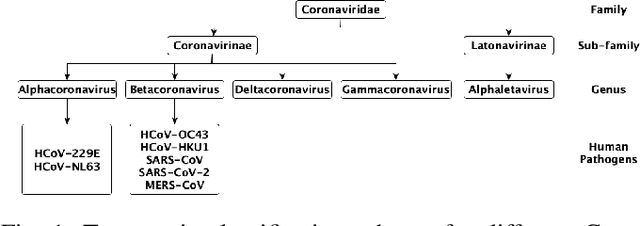
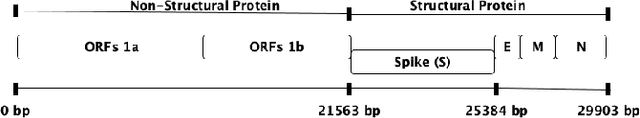
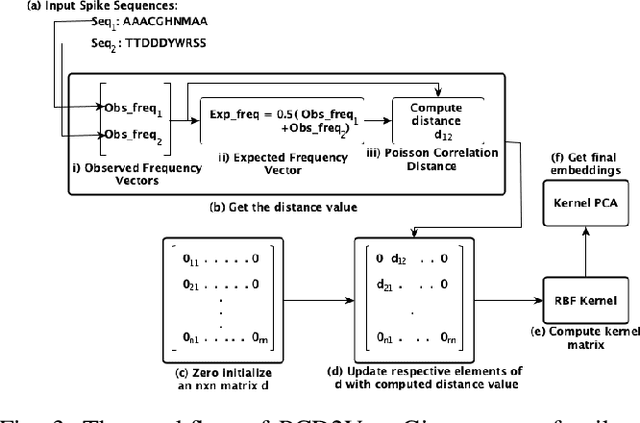
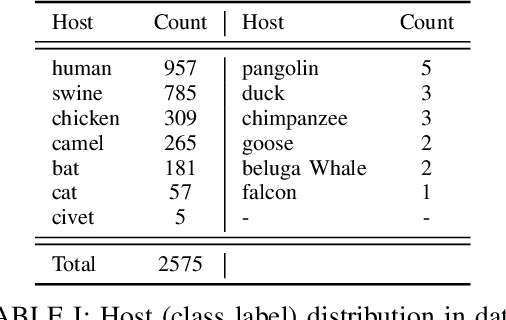
Coronaviruses are membrane-enveloped, non-segmented positive-strand RNA viruses belonging to the Coronaviridae family. Various animal species, mainly mammalian and avian, are severely infected by various coronaviruses, causing serious concerns like the recent pandemic (COVID-19). Therefore, building a deeper understanding of these viruses is essential to devise prevention and mitigation mechanisms. In the Coronavirus genome, an essential structural region is the spike region, and it's responsible for attaching the virus to the host cell membrane. Therefore, the usage of only the spike protein, instead of the full genome, provides most of the essential information for performing analyses such as host classification. In this paper, we propose a novel method for predicting the host specificity of coronaviruses by analyzing spike protein sequences from different viral subgenera and species. Our method involves using the Poisson correction distance to generate a distance matrix, followed by using a radial basis function (RBF) kernel and kernel principal component analysis (PCA) to generate a low-dimensional embedding. Finally, we apply classification algorithms to the low-dimensional embedding to generate the resulting predictions of the host specificity of coronaviruses. We provide theoretical proofs for the non-negativity, symmetry, and triangle inequality properties of the Poisson correction distance metric, which are important properties in a machine-learning setting. By encoding the spike protein structure and sequences using this comprehensive approach, we aim to uncover hidden patterns in the biological sequences to make accurate predictions about host specificity. Finally, our classification results illustrate that our method can achieve higher predictive accuracy and improve performance over existing baselines.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge