PC-Conv: Unifying Homophily and Heterophily with Two-fold Filtering
Paper and Code
Dec 22, 2023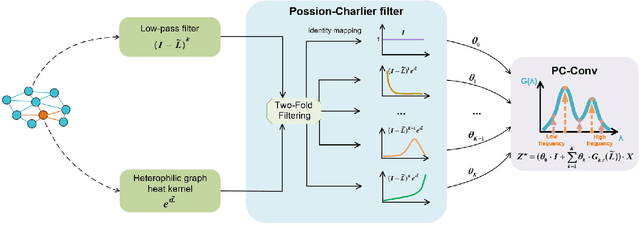
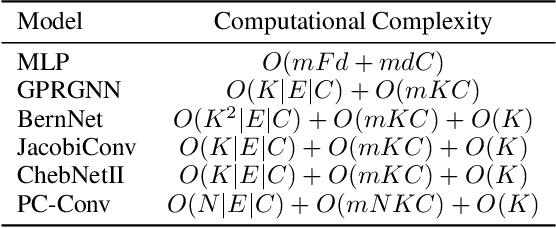
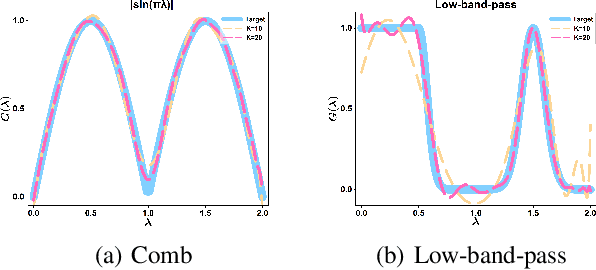
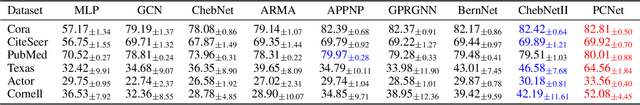
Recently, many carefully crafted graph representation learning methods have achieved impressive performance on either strong heterophilic or homophilic graphs, but not both. Therefore, they are incapable of generalizing well across real-world graphs with different levels of homophily. This is attributed to their neglect of homophily in heterophilic graphs, and vice versa. In this paper, we propose a two-fold filtering mechanism to extract homophily in heterophilic graphs and vice versa. In particular, we extend the graph heat equation to perform heterophilic aggregation of global information from a long distance. The resultant filter can be exactly approximated by the Possion-Charlier (PC) polynomials. To further exploit information at multiple orders, we introduce a powerful graph convolution PC-Conv and its instantiation PCNet for the node classification task. Compared with state-of-the-art GNNs, PCNet shows competitive performance on well-known homophilic and heterophilic graphs. Our implementation is available at https://github.com/uestclbh/PC-Conv.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge