Passive Reaction Analysis for Grasp Stability
Paper and Code
Jan 19, 2018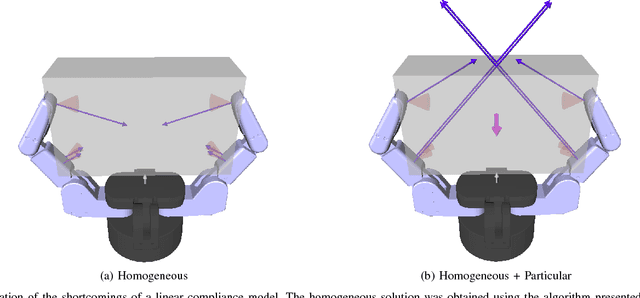
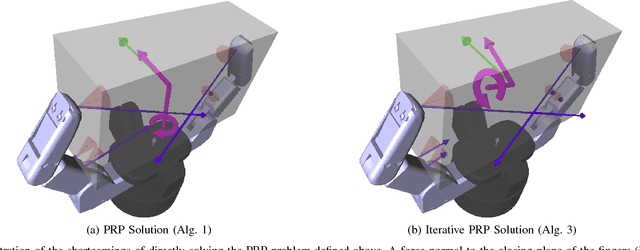
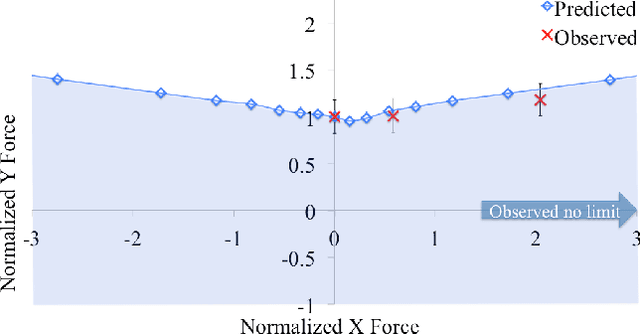
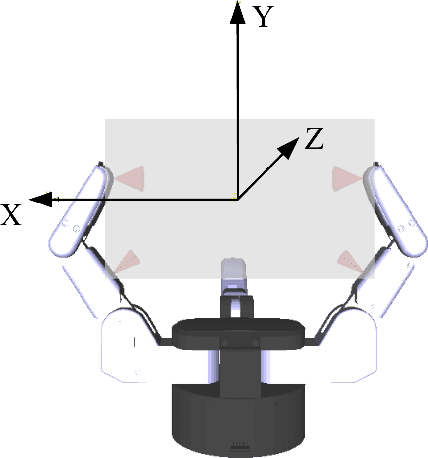
In this paper we focus on the following problem in multi-fingered robotic grasping: assuming that an external wrench is being applied to a grasped object, will the contact forces between the hand and the object, as well as the hand joints, respond in such a way as to preserve quasi-static equilibrium? In particular, we assume that there is no change in the joint torques being actively exerted by the motors; any change in contact forces and joint torques is due exclusively to passive effects arising in response to the external disturbance. Such passive effects include for example joints that are driven by highly geared motors (a common occurence in practice) and thus do not back drive in response to external torques. To account for non- linear phenomena encountered in such cases, and which existing methods do not consider, we formulate the problem as a mixed integer program used in the inner loop of an iterative solver. We present evidence showing that this formulation captures important effects for assessing the stability of a grasp employing some of the most commonly used actuation mechanisms.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge