On Centroidal Dynamics and Integrability of Average Angular Velocity
Paper and Code
Jan 10, 2017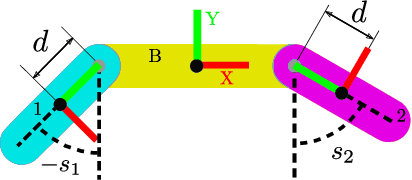
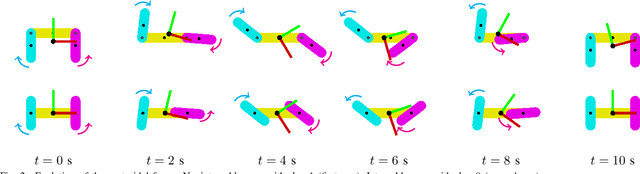
In the literature on robotics and multibody dynamics, the concept of average angular velocity has received considerable attention in recent years. We address the question of whether the average angular velocity defines an orientation framethat depends only on the current robot configuration and provide a simple algebraic condition to check whether this holds. In the language of geometric mechanics, this condition corresponds to requiring the flatness of the mechanical connection associated to the robotic system. Here, however, we provide both a reinterpretation and a proof of this result accessible to readers with a background in rigid body kinematics and multibody dynamics but not necessarily acquainted with differential geometry, still providing precise links to the geometric mechanics literature. This should help spreading the algebraic condition beyond the scope of geometric mechanics,contributing to a proper utilization and understanding of the concept of average angular velocity.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge