Neural Ordinary Differential Equations for Intervention Modeling
Paper and Code
Oct 16, 2020
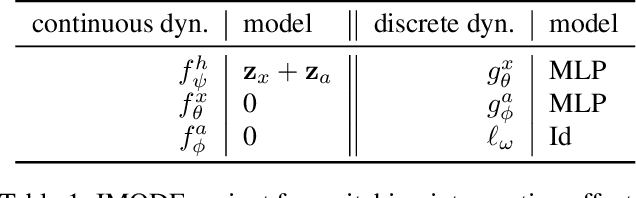
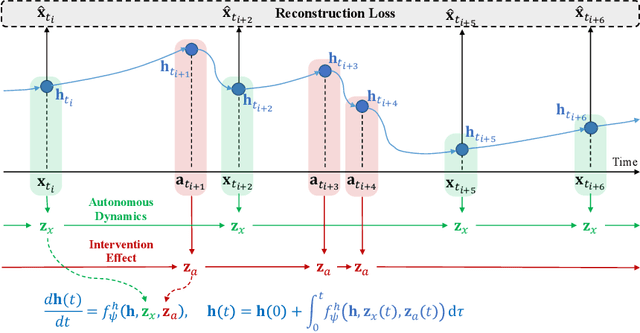
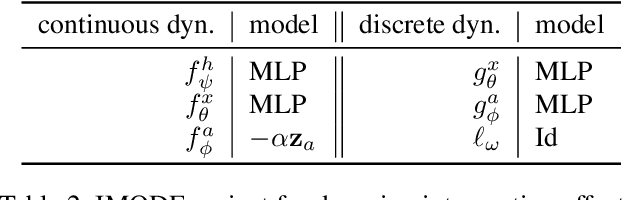
By interpreting the forward dynamics of the latent representation of neural networks as an ordinary differential equation, Neural Ordinary Differential Equation (Neural ODE) emerged as an effective framework for modeling a system dynamics in the continuous time domain. However, real-world systems often involves external interventions that cause changes in the system dynamics such as a moving ball coming in contact with another ball, or such as a patient being administered with particular drug. Neural ODE and a number of its recent variants, however, are not suitable for modeling such interventions as they do not properly model the observations and the interventions separately. In this paper, we propose a novel neural ODE-based approach (IMODE) that properly model the effect of external interventions by employing two ODE functions to separately handle the observations and the interventions. Using both synthetic and real-world time-series datasets involving interventions, our experimental results consistently demonstrate the superiority of IMODE compared to existing approaches.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge