Multi-task Supervised Learning via Cross-learning
Paper and Code
Oct 30, 2020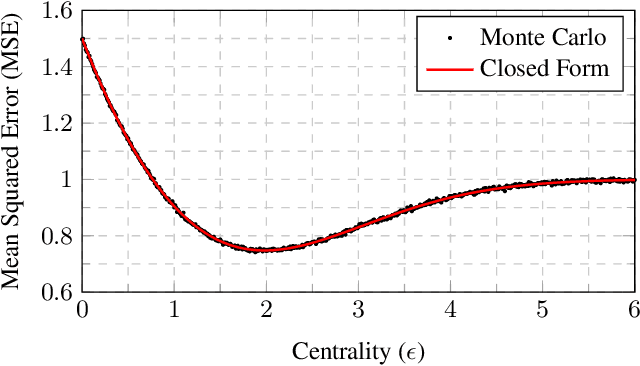

In this paper we consider a problem known as multi-task learning, consisting of fitting a set of classifier or regression functions intended for solving different tasks. In our novel formulation, we couple the parameters of these functions, so that they learn in their task specific domains while staying close to each other. This facilitates cross-fertilization in which data collected across different domains help improving the learning performance at each other task. First, we present a simplified case in which the goal is to estimate the means of two Gaussian variables, for the purpose of gaining some insights on the advantage of the proposed cross-learning strategy. Then we provide a stochastic projected gradient algorithm to perform cross-learning over a generic loss function. If the number of parameters is large, then the projection step becomes computationally expensive. To avoid this situation, we derive a primal-dual algorithm that exploits the structure of the dual problem, achieving a formulation whose complexity only depends on the number of tasks. Preliminary numerical experiments for image classification by neural networks trained on a dataset divided in different domains corroborate that the cross-learned function outperforms both the task-specific and the consensus approaches.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge