Multi-Agent Path Planning with Asymmetric Interactions In Tight Spaces
Paper and Code
Apr 01, 2022
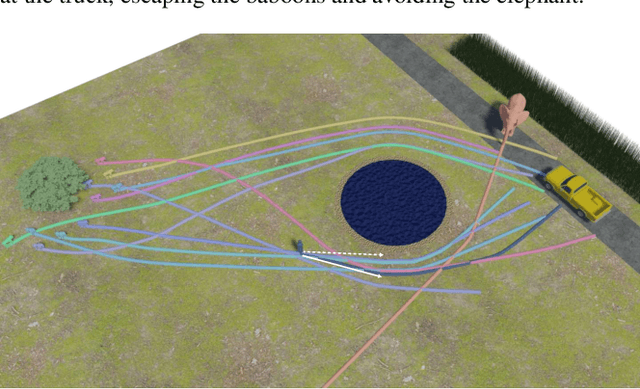


By starting with the assumption that motion is fundamentally a decision making problem, we use the world-line concept from Special Relativity as the inspiration for a novel multi-agent path planning method. We have identified a particular set of problems that have so far been overlooked by previous works. We present our solution for the global path planning problem for each agent and ensure smooth local collision avoidance for each pair of agents in the scene. We accomplish this by modeling the trajectories of the agents through 2D space and time as curves in 3D. Global path planning is solved using a modified Djikstra's algorithm to ensure that initial trajectories for agents do not intersect. We then solve for smooth local trajectories using a quasi-Newton interior point solver, providing the trajectory curves with a radius to turn them into rods. Subsequently, resolving collision of the rods ensures that no two agents are in the same spatial position at the same time. This space-time formulation allows us to simulate previously ignored phenomena such as highly asymmetric interactions in very constrained environments. It also provides a solution for scenes with unnaturally symmetric agent alignments without the need for jittering agent positions or velocities.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge