Modeling the average shortest path length in growth of word-adjacency networks
Paper and Code
Mar 06, 2015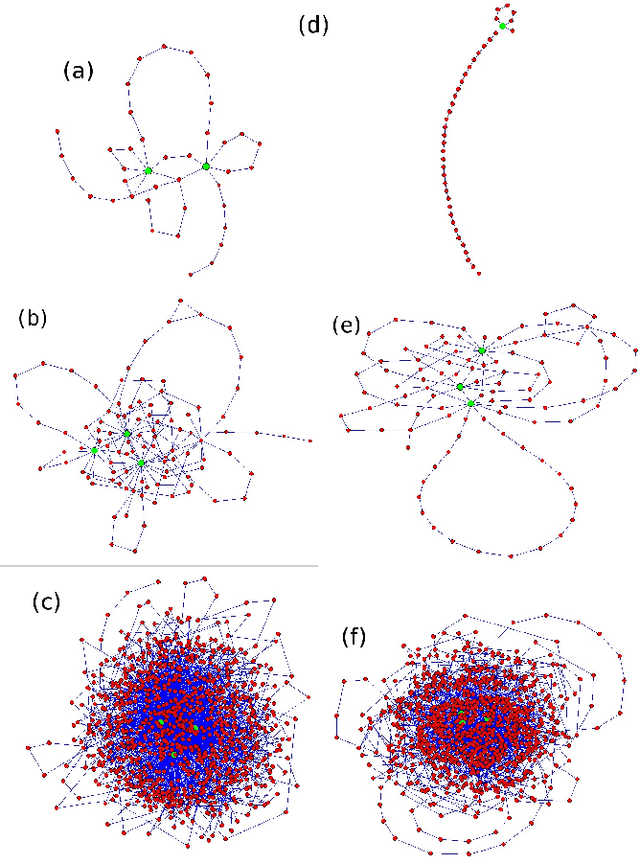
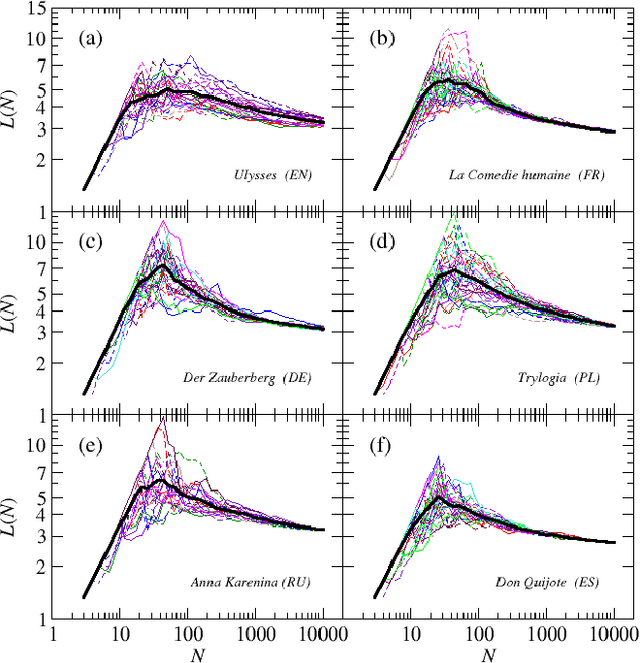
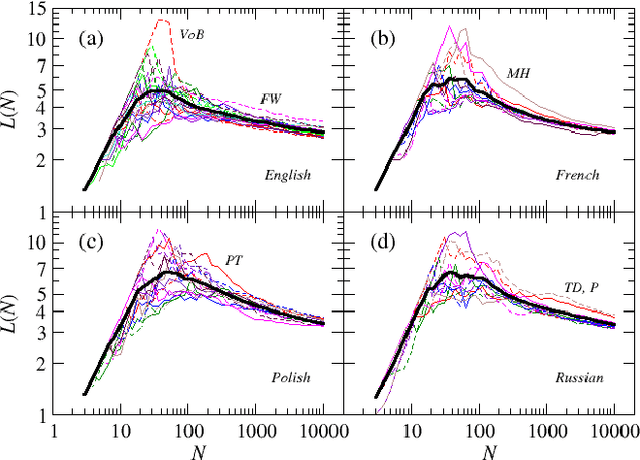
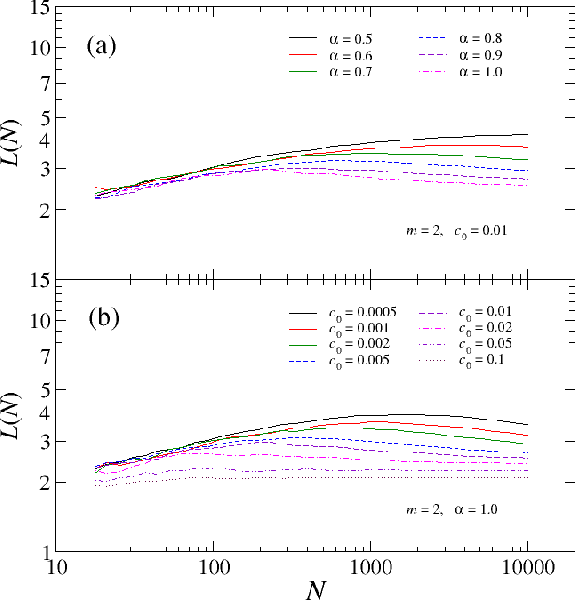
We investigate properties of evolving linguistic networks defined by the word-adjacency relation. Such networks belong to the category of networks with accelerated growth but their shortest path length appears to reveal the network size dependence of different functional form than the ones known so far. We thus compare the networks created from literary texts with their artificial substitutes based on different variants of the Dorogovtsev-Mendes model and observe that none of them is able to properly simulate the novel asymptotics of the shortest path length. Then, we identify the local chain-like linear growth induced by grammar and style as a missing element in this model and extend it by incorporating such effects. It is in this way that a satisfactory agreement with the empirical result is obtained.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge