Möbius Photogrammetry

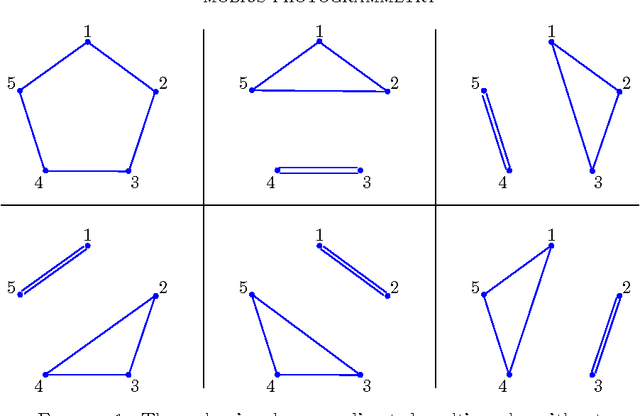
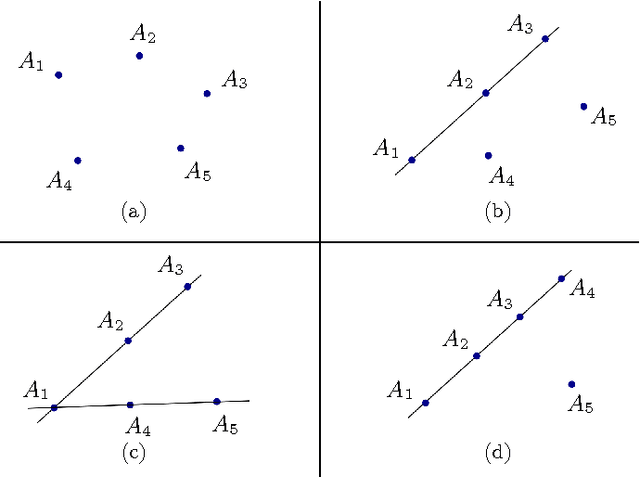
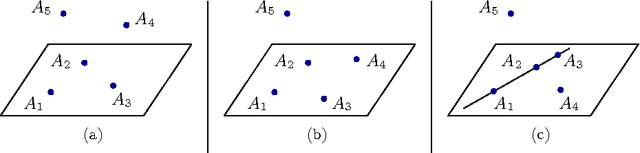
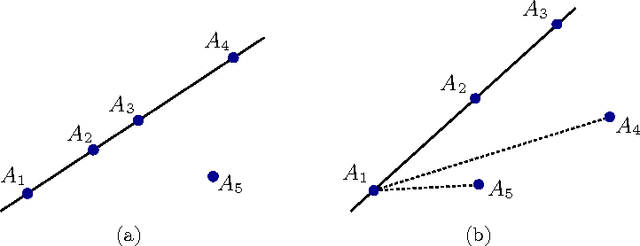
Motivated by results on the mobility of mechanical devices called pentapods, this paper deals with a mathematically freestanding problem, which we call M\"obius Photogrammetry. Unlike traditional photogrammetry, which tries to recover a set of points in three-dimensional space from a finite set of central projection, we consider the problem of reconstructing a vector of points in $\mathbb{R}^3$ starting from its orthogonal parallel projections. Moreover, we assume that we have partial information about these projections, namely that we know them only up to M\"obius transformations. The goal in this case is to understand to what extent we can reconstruct the starting set of points, and to prove that the result can be achieved if we allow some uncertainties in the answer. Eventually, the techniques developed in the paper allow us to show that for a pentapod with mobility at least two either some anchor points are collinear, or platform and base are similar, or they are planar and affine equivalent.