Logical Conditional Preference Theories
Paper and Code
Apr 24, 2015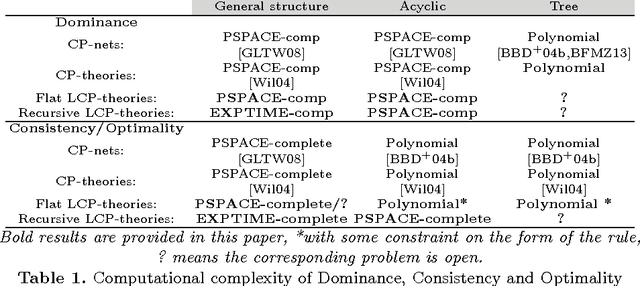
CP-nets represent the dominant existing framework for expressing qualitative conditional preferences between alternatives, and are used in a variety of areas including constraint solving. Over the last fifteen years, a significant literature has developed exploring semantics, algorithms, implementation and use of CP-nets. This paper introduces a comprehensive new framework for conditional preferences: logical conditional preference theories (LCP theories). To express preferences, the user specifies arbitrary (constraint) Datalog programs over a binary ordering relation on outcomes. We show how LCP theories unify and generalize existing conditional preference proposals, and leverage the rich semantic, algorithmic and implementation frameworks of Datalog.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge