Linear Convergence of Proximal Gradient Algorithm with Extrapolation for a Class of Nonconvex Nonsmooth Minimization Problems
Paper and Code
Aug 01, 2016
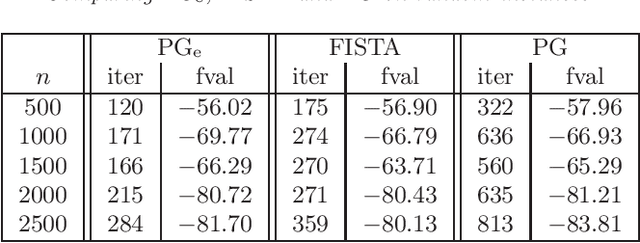


In this paper, we study the proximal gradient algorithm with extrapolation for minimizing the sum of a Lipschitz differentiable function and a proper closed convex function. Under the error bound condition used in [19] for analyzing the convergence of the proximal gradient algorithm, we show that there exists a threshold such that if the extrapolation coefficients are chosen below this threshold, then the sequence generated converges $R$-linearly to a stationary point of the problem. Moreover, the corresponding sequence of objective values is also $R$-linearly convergent. In addition, the threshold reduces to $1$ for convex problems and, as a consequence, we obtain the $R$-linear convergence of the sequence generated by FISTA with fixed restart. Finally, we present some numerical experiments to illustrate our results.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge