Instantaneous GNSS Ambiguity Resolution and Attitude Determination via Riemannian Manifold Optimization
Paper and Code
May 20, 2022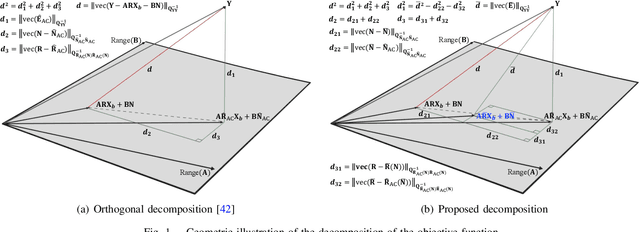
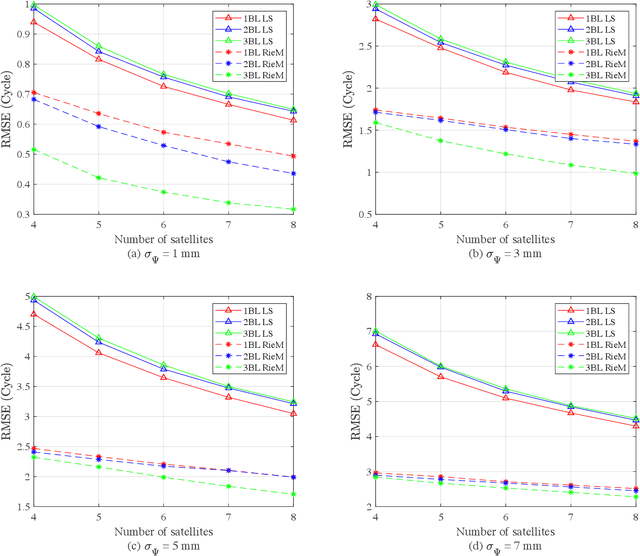
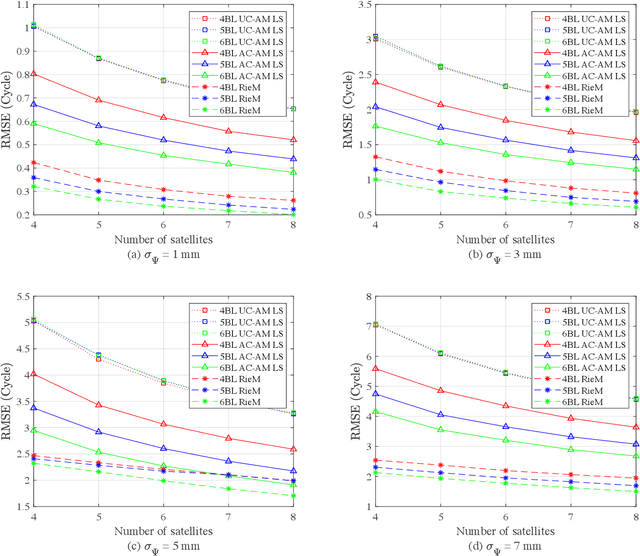

We present an ambiguity resolution method for Global Navigation Satellite System (GNSS)-based attitude determination. A GNSS attitude model with nonlinear constraints is used to rigorously incorporate a priori information. Given the characteristics of the employed nonlinear constraints, we formulate GNSS attitude determination as an optimization problem on a manifold. Then, Riemannian manifold optimization algorithms are utilized to aid ambiguity resolution based on a proposed decomposition of the objective function. The application of manifold geometry enables high-quality float solutions that are critical to reinforcing search-based integer ambiguity resolution in terms of efficiency, availability, and reliability. The proposed approach is characterized by a low computational complexity and a high probability of resolving the ambiguities correctly. The performance of the proposed ambiguity resolution method is tested through a series of simulations and real experiments. Comparisons with the principal benchmarks indicate the superiority of the proposed method as reflected by the high ambiguity resolution success rates.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge