Hierarchical Representations with Poincaré Variational Auto-Encoders

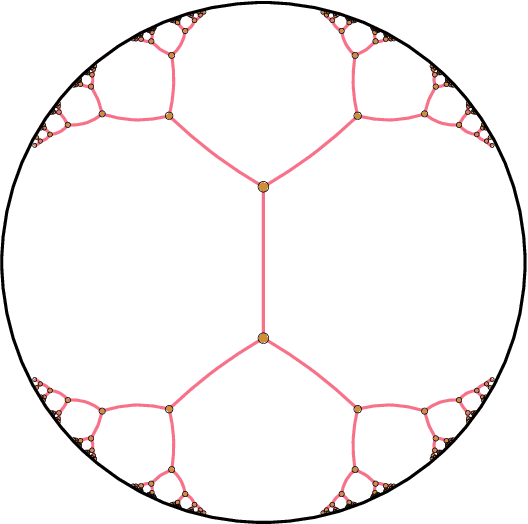
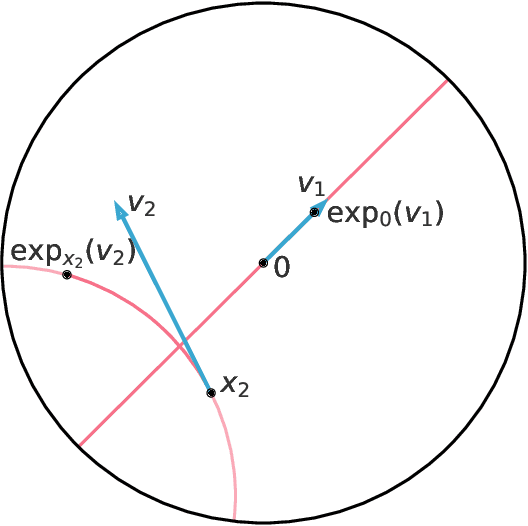
The Variational Auto-Encoder (VAE) model has become widely popular as a way to learn at once a generative model and embeddings for observations living in a high-dimensional space. In the real world, many such observations may be assumed to be hierarchically structured, such as living organisms data which are related through the evolutionary tree. Also, it has been theoretically and empirically shown that data with hierarchical structure can efficiently be embedded in hyperbolic spaces. We therefore endow the VAE with a hyperbolic geometry and empirically show that it can better generalise to unseen data than its Euclidean counterpart, and can qualitatively recover the hierarchical structure.