Generalized Nonlinear and Finsler Geometry for Robotics

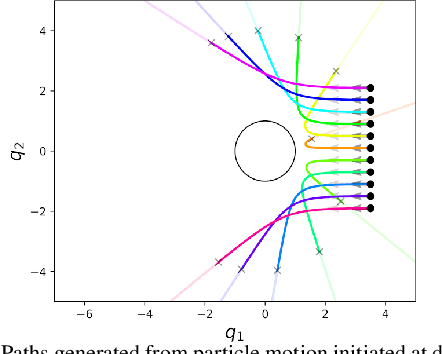
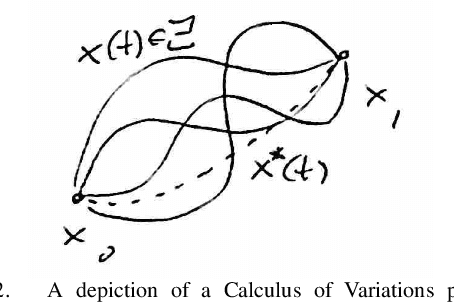
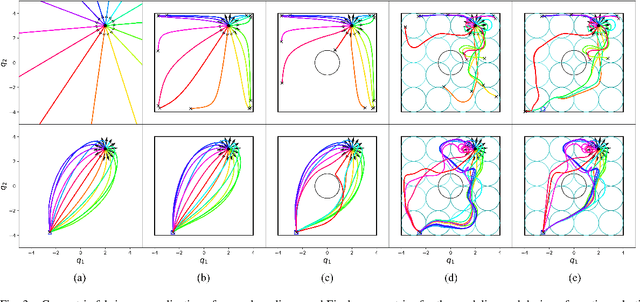
Robotics research has found numerous important applications of Riemannian geometry. Despite that, the concept remain challenging to many roboticists because the background material is complex and strikingly foreign. Beyond Riemannian geometry, there are many natural generalizations in the mathematical literature---areas such as Finsler geometry and spray geometry---but those generalizations are largely inaccessible, and as a result there remain few applications within robotics. This paper presents a re-derivation of spray and Finsler geometries, critical for the development of our recent work on geometric fabrics, which builds the ideas from familiar concepts in advanced calculus and the calculus of variations. We focus on the pragmatic and calculable results, avoiding the use of tensor notation to appeal to a broader audience and emphasizing geometric path consistency over ideas around connections and curvature. It is our hope that they will contribute to an increased understanding generalized nonlinear, and even classical Riemannian, geometry within the robotics community and inspire future research into new applications.