Encoding Temporal Statistical-space Priors via Augmented Representation
Paper and Code
Feb 03, 2024

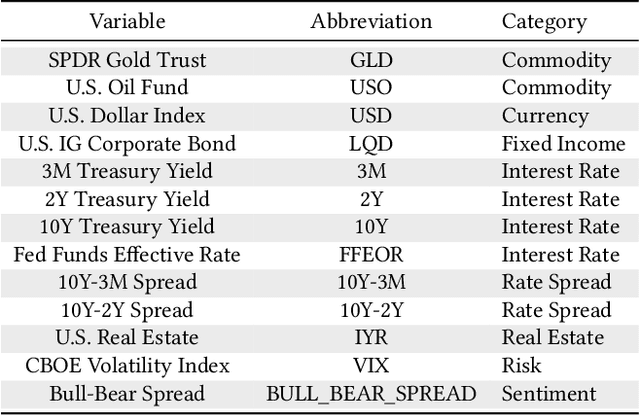

Modeling time series data remains a pervasive issue as the temporal dimension is inherent to numerous domains. Despite significant strides in time series forecasting, high noise-to-signal ratio, non-normality, non-stationarity, and lack of data continue challenging practitioners. In response, we leverage a simple representation augmentation technique to overcome these challenges. Our augmented representation acts as a statistical-space prior encoded at each time step. In response, we name our method Statistical-space Augmented Representation (SSAR). The underlying high-dimensional data-generating process inspires our representation augmentation. We rigorously examine the empirical generalization performance on two data sets with two downstream temporal learning algorithms. Our approach significantly beats all five up-to-date baselines. Moreover, the highly modular nature of our approach can easily be applied to various settings. Lastly, fully-fledged theoretical perspectives are available throughout the writing for a clear and rigorous understanding.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge