Emergence of a stochastic resonance in machine learning
Paper and Code
Nov 15, 2022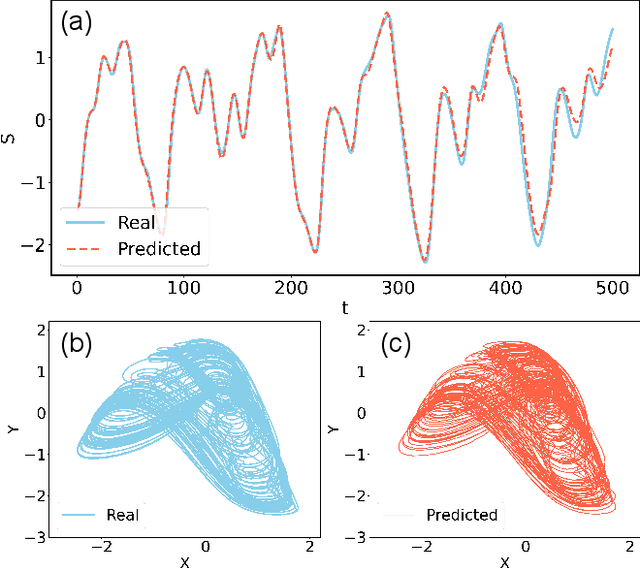
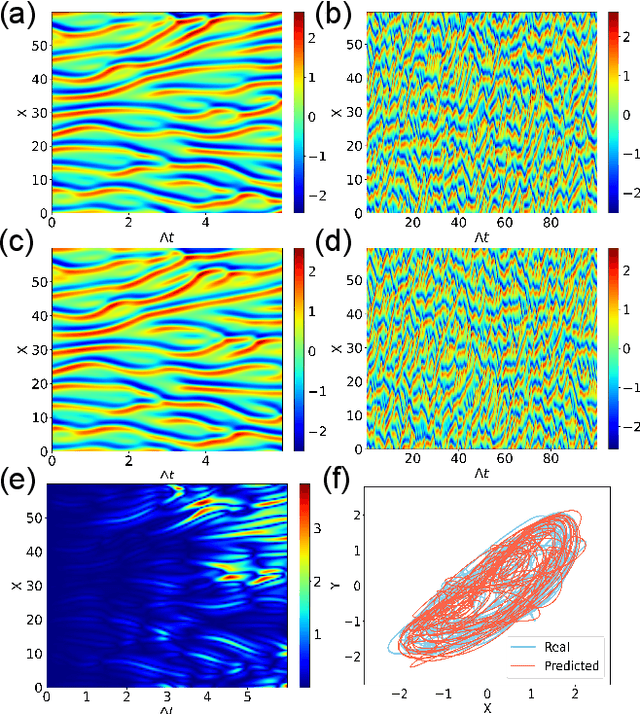
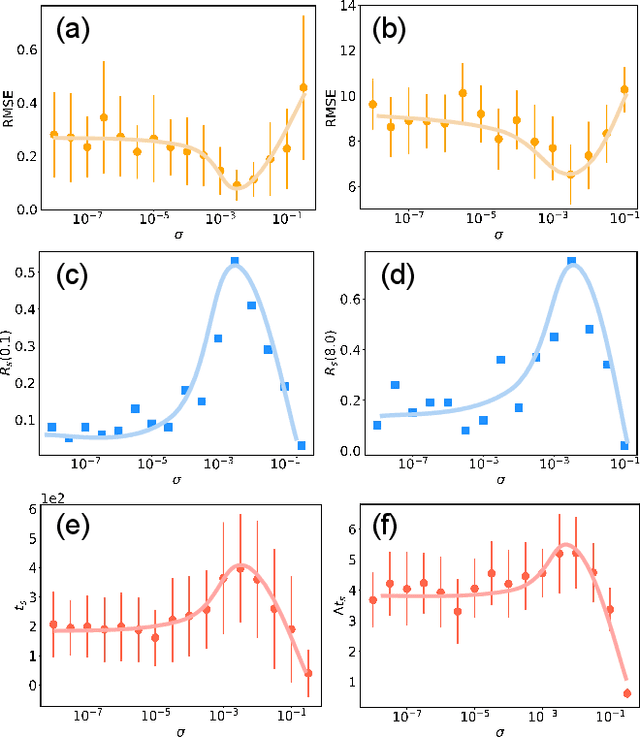
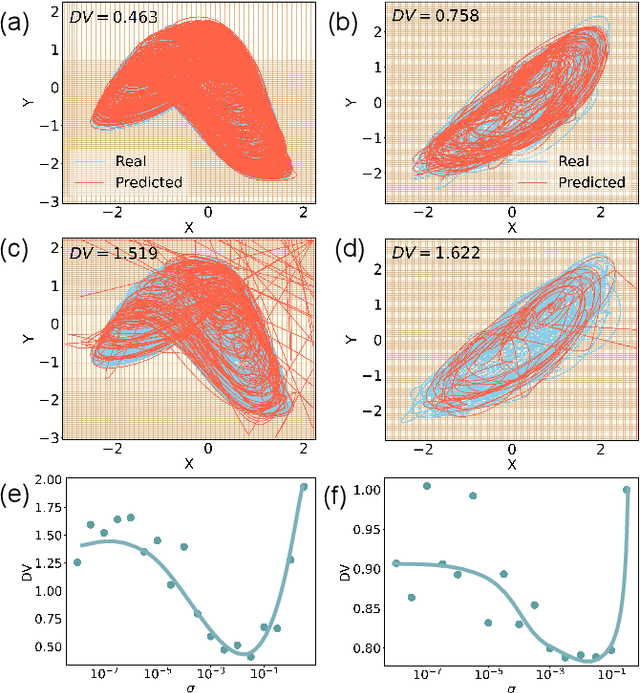
Can noise be beneficial to machine-learning prediction of chaotic systems? Utilizing reservoir computers as a paradigm, we find that injecting noise to the training data can induce a stochastic resonance with significant benefits to both short-term prediction of the state variables and long-term prediction of the attractor of the system. A key to inducing the stochastic resonance is to include the amplitude of the noise in the set of hyperparameters for optimization. By so doing, the prediction accuracy, stability and horizon can be dramatically improved. The stochastic resonance phenomenon is demonstrated using two prototypical high-dimensional chaotic systems.
* 7 pages, 4 figures
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge