Denoising Linear Models with Permuted Data
Paper and Code
Apr 24, 2017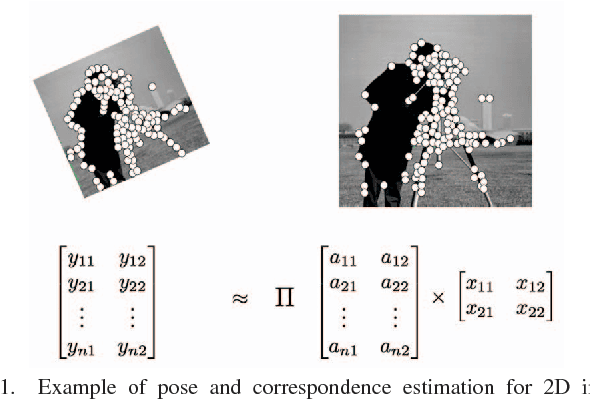

The multivariate linear regression model with shuffled data and additive Gaussian noise arises in various correspondence estimation and matching problems. Focusing on the denoising aspect of this problem, we provide a characterization the minimax error rate that is sharp up to logarithmic factors. We also analyze the performance of two versions of a computationally efficient estimator, and establish their consistency for a large range of input parameters. Finally, we provide an exact algorithm for the noiseless problem and demonstrate its performance on an image point-cloud matching task. Our analysis also extends to datasets with outliers.
* To appear in part at ISIT 2017, Aachen
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge