Deep Metric Learning with Spherical Embedding
Paper and Code
Nov 05, 2020
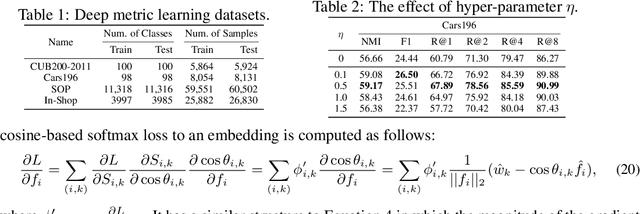
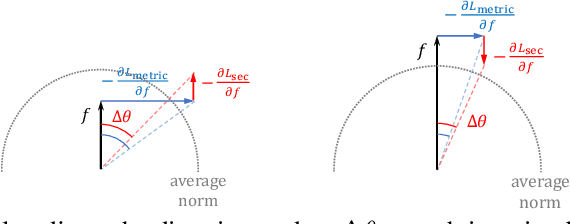
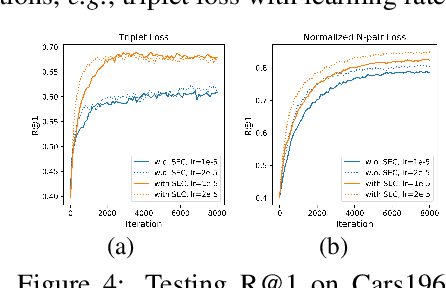
Deep metric learning has attracted much attention in recent years, due to seamlessly combining the distance metric learning and deep neural network. Many endeavors are devoted to design different pair-based angular loss functions, which decouple the magnitude and direction information for embedding vectors and ensure the training and testing measure consistency. However, these traditional angular losses cannot guarantee that all the sample embeddings are on the surface of the same hypersphere during the training stage, which would result in unstable gradient in batch optimization and may influence the quick convergence of the embedding learning. In this paper, we first investigate the effect of the embedding norm for deep metric learning with angular distance, and then propose a spherical embedding constraint (SEC) to regularize the distribution of the norms. SEC adaptively adjusts the embeddings to fall on the same hypersphere and performs more balanced direction update. Extensive experiments on deep metric learning, face recognition, and contrastive self-supervised learning show that the SEC-based angular space learning strategy significantly improves the performance of the state-of-the-art.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge