Convexity Shape Prior for Level Set based Image Segmentation Method
Paper and Code
May 22, 2018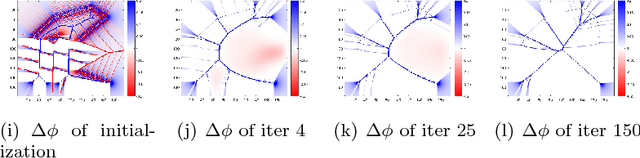
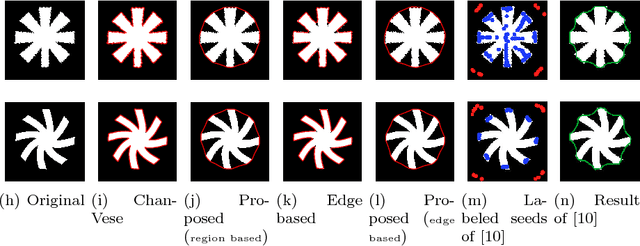
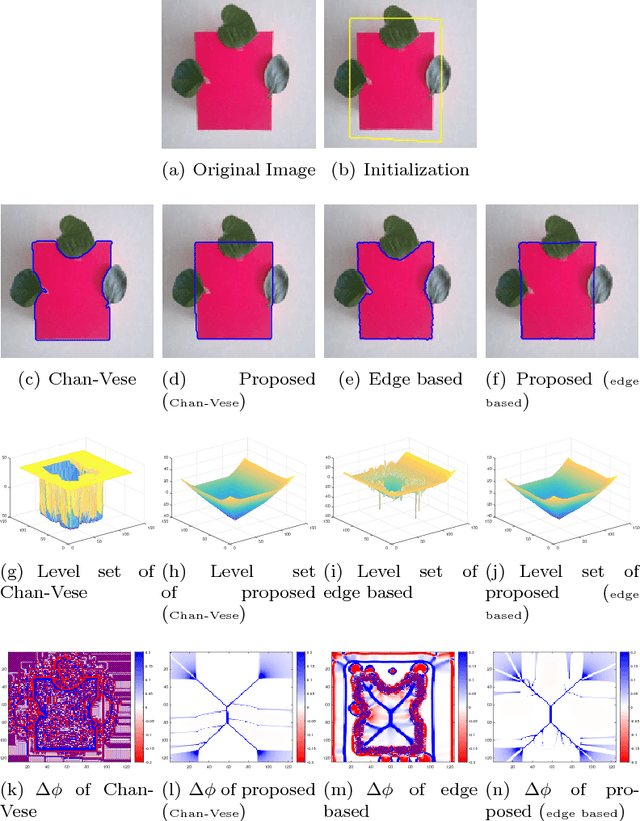
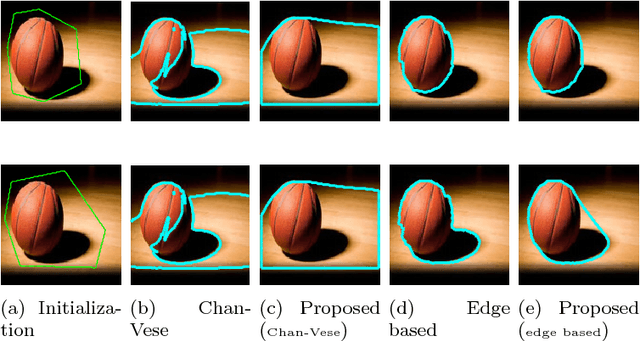
We propose a geometric convexity shape prior preservation method for variational level set based image segmentation methods. Our method is built upon the fact that the level set of a convex signed distanced function must be convex. This property enables us to transfer a complicated geometrical convexity prior into a simple inequality constraint on the function. An active set based Gauss-Seidel iteration is used to handle this constrained minimization problem to get an efficient algorithm. We apply our method to region and edge based level set segmentation models including Chan-Vese (CV) model with guarantee that the segmented region will be convex. Experimental results show the effectiveness and quality of the proposed model and algorithm.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge