As Easy as ABC: Adaptive Binning Coincidence Test for Uniformity Testing
Paper and Code
Oct 12, 2021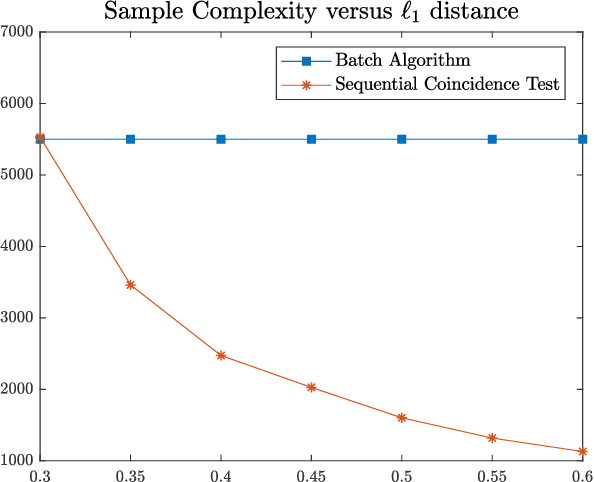
We consider the problem of uniformity testing of Lipschitz continuous distributions with bounded support. The alternative hypothesis is a composite set of Lipschitz continuous distributions that are at least $\varepsilon$ away in $\ell_1$ distance from the uniform distribution. We propose a sequential test that adapts to the unknown distribution under the alternative hypothesis. Referred to as the Adaptive Binning Coincidence (ABC) test, the proposed strategy adapts in two ways. First, it partitions the set of alternative distributions into layers based on their distances to the uniform distribution. It then sequentially eliminates the alternative distributions layer by layer in decreasing distance to the uniform, and subsequently takes advantage of favorable situations of a distant alternative by exiting early. Second, it adapts, across layers of the alternative distributions, the resolution level of the discretization for computing the coincidence statistic. The farther away the layer is from the uniform, the coarser the discretization is needed for eliminating/exiting this layer. It thus exits both early in the detection process and quickly by using a lower resolution to take advantage of favorable alternative distributions. The ABC test builds on a novel sequential coincidence test for discrete distributions, which is of independent interest. We establish the sample complexity of the proposed tests as well as a lower bound.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge