An Efficient Algorithm for the Piecewise-Smooth Model with Approximately Explicit Solutions

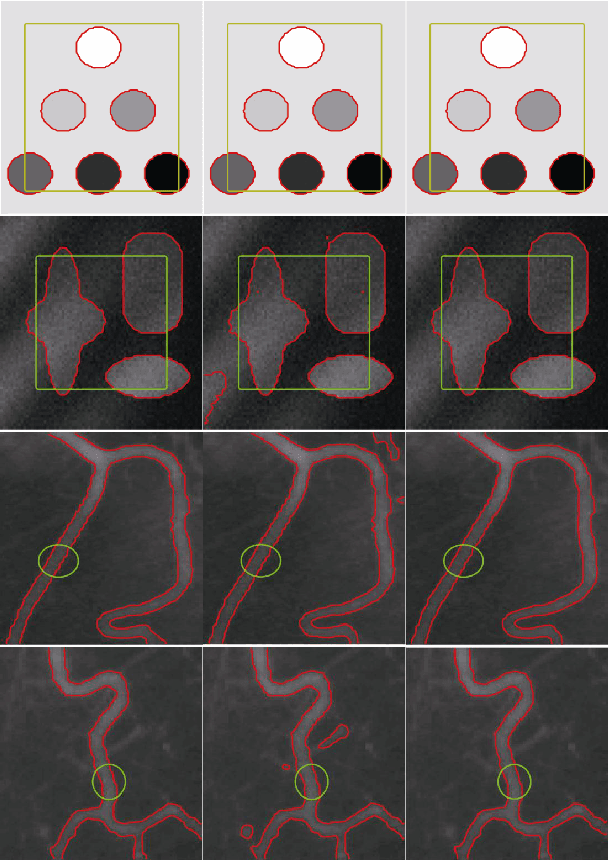
This paper presents an efficient approach to image segmentation that approximates the piecewise-smooth (PS) functional in [12] with explicit solutions. By rendering some rational constraints on the initial conditions and the final solutions of the PS functional, we propose two novel formulations which can be approximated to be the explicit solutions of the evolution partial differential equations (PDEs) of the PS model, in which only one PDE needs to be solved efficiently. Furthermore, an energy term that regularizes the level set function to be a signed distance function is incorporated into our evolution formulation, and the time-consuming re-initialization is avoided. Experiments on synthetic and real images show that our method is more efficient than both the PS model and the local binary fitting (LBF) model [4], while having similar segmentation accuracy as the LBF model.