Jump-Start Reinforcement Learning with Self-Evolving Priors for Extreme Monopedal Locomotion

Reinforcement learning (RL) has shown great potential in enabling quadruped robots to perform agile locomotion. However, directly training policies to simultaneously handle dual extreme challenges, i.e., extreme underactuation and extreme terrains, as in monopedal hopping tasks, remains highly challenging due to unstable early-stage interactions and unreliable reward feedback. To address this, we propose JumpER (jump-start reinforcement learning via self-evolving priors), an RL training framework that structures policy learning into multiple stages of increasing complexity. By dynamically generating self-evolving priors through iterative bootstrapping of previously learned policies, JumpER progressively refines and enhances guidance, thereby stabilizing exploration and policy optimization without relying on external expert priors or handcrafted reward shaping. Specifically, when integrated with a structured three-stage curriculum that incrementally evolves action modality, observation space, and task objective, JumpER enables quadruped robots to achieve robust monopedal hopping on unpredictable terrains for the first time. Remarkably, the resulting policy effectively handles challenging scenarios that traditional methods struggle to conquer, including wide gaps up to 60 cm, irregularly spaced stairs, and stepping stones with distances varying from 15 cm to 35 cm. JumpER thus provides a principled and scalable approach for addressing locomotion tasks under the dual challenges of extreme underactuation and extreme terrains.

Canonical Form of Datatic Description in Control Systems

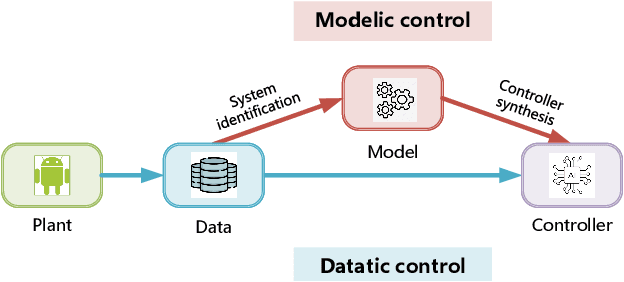
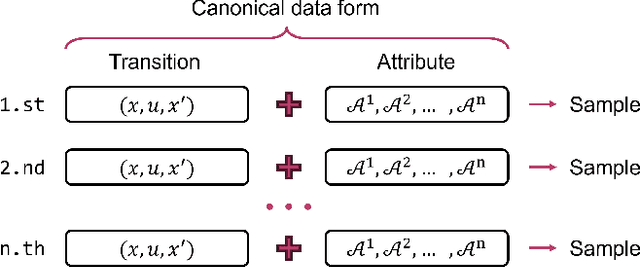
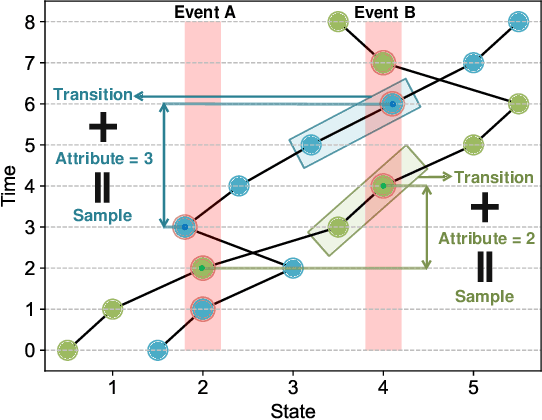
The design of feedback controllers is undergoing a paradigm shift from modelic (i.e., model-driven) control to datatic (i.e., data-driven) control. Canonical form of state space model is an important concept in modelic control systems, exemplified by Jordan form, controllable form and observable form, whose purpose is to facilitate system analysis and controller synthesis. In the realm of datatic control, there is a notable absence in the standardization of data-based system representation. This paper for the first time introduces the concept of canonical data form for the purpose of achieving more effective design of datatic controllers. In a control system, the data sample in canonical form consists of a transition component and an attribute component. The former encapsulates the plant dynamics at the sampling time independently, which is a tuple containing three elements: a state, an action and their corresponding next state. The latter describes one or some artificial characteristics of the current sample, whose calculation must be performed in an online manner. The attribute of each sample must adhere to two requirements: (1) causality, ensuring independence from any future samples; and (2) locality, allowing dependence on historical samples but constrained to a finite neighboring set. The purpose of adding attribute is to offer some kinds of benefits for controller design in terms of effectiveness and efficiency. To provide a more close-up illustration, we present two canonical data forms: temporal form and spatial form, and demonstrate their advantages in reducing instability and enhancing training efficiency in two datatic control systems.