Yi-Chun Xu
Ant Colony Algorithm for the Weighted Item Layout Optimization Problem
Jan 24, 2010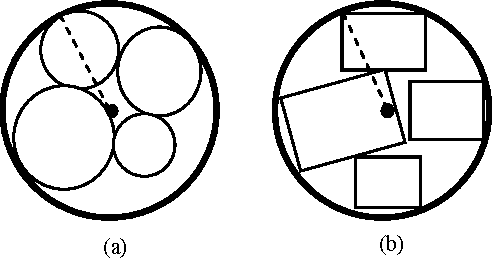
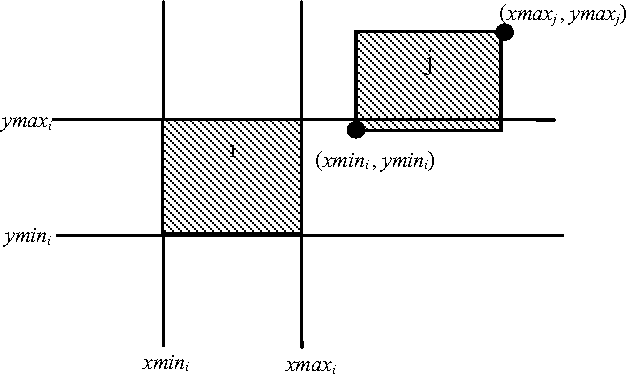
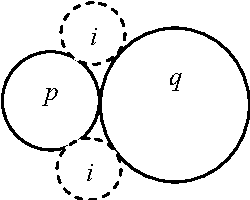
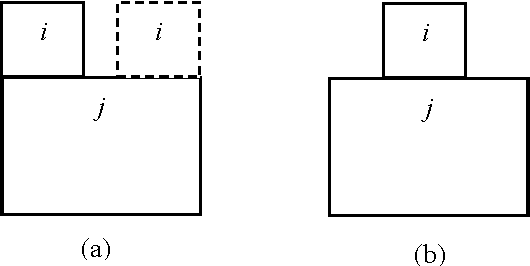
Abstract:This paper discusses the problem of placing weighted items in a circular container in two-dimensional space. This problem is of great practical significance in various mechanical engineering domains, such as the design of communication satellites. Two constructive heuristics are proposed, one for packing circular items and the other for packing rectangular items. These work by first optimizing object placement order, and then optimizing object positioning. Based on these heuristics, an ant colony optimization (ACO) algorithm is described to search first for optimal positioning order, and then for the optimal layout. We describe the results of numerical experiments, in which we test two versions of our ACO algorithm alongside local search methods previously described in the literature. Our results show that the constructive heuristic-based ACO performs better than existing methods on larger problem instances.
Simulated annealing for weighted polygon packing
Sep 29, 2008
Abstract:In this paper we present a new algorithm for a layout optimization problem: this concerns the placement of weighted polygons inside a circular container, the two objectives being to minimize imbalance of mass and to minimize the radius of the container. This problem carries real practical significance in industrial applications (such as the design of satellites), as well as being of significant theoretical interest. Previous work has dealt with circular or rectangular objects, but here we deal with the more realistic case where objects may be represented as polygons and the polygons are allowed to rotate. We present a solution based on simulated annealing and first test it on instances with known optima. Our results show that the algorithm obtains container radii that are close to optimal. We also compare our method with existing algorithms for the (special) rectangular case. Experimental results show that our approach out-performs these methods in terms of solution quality.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge