Xiefeng Cheng
Nonconvex Nonsmooth Low-Rank Minimization for Generalized Image Compressed Sensing via Group Sparse Representation
Nov 18, 2019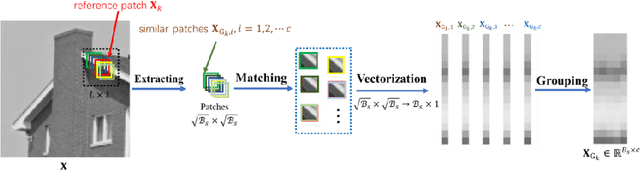
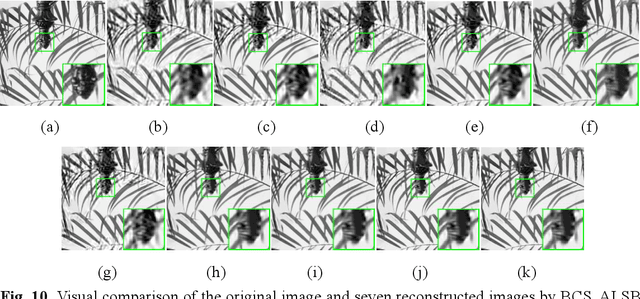
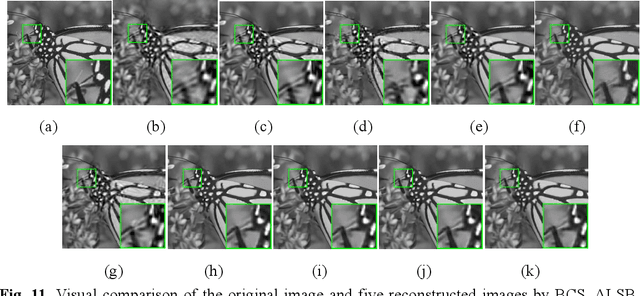
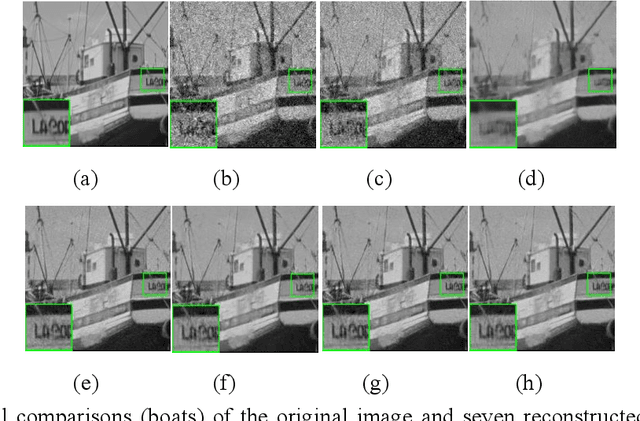
Abstract:Group sparse representation (GSR) based method has led to great successes in various image recovery tasks, which can be converted into a low-rank matrix minimization problem. As a widely used surrogate function of low-rank, the nuclear norm based convex surrogate usually leads to over-shrinking problem, since the standard soft-thresholding operator shrinks all singular values equally. To improve traditional sparse representation based image compressive sensing (CS) performance, we propose a generalized CS framework based on GSR model, leading to a nonconvex nonsmooth low-rank minimization problem. The popular L_2-norm and M-estimator are employed for standard image CS and robust CS problem to fit the data respectively. For the better approximation of the rank of group-matrix, a family of nuclear norms are employed to address the over-shrinking problem. Moreover, we also propose a flexible and effective iteratively-weighting strategy to control the weighting and contribution of each singular value. Then we develop an iteratively reweighted nuclear norm algorithm for our generalized framework via an alternating direction method of multipliers framework, namely, GSR-ADMM-IRNN. Experimental results demonstrate that our proposed CS framework can achieve favorable reconstruction performance compared with current state-of-the-art methods and the RCS framework can suppress the outliers effectively.
Generalized Rank Minimization based Group Sparse Coding for Low-level Image Restoration via Dictionary Learning
Jul 13, 2019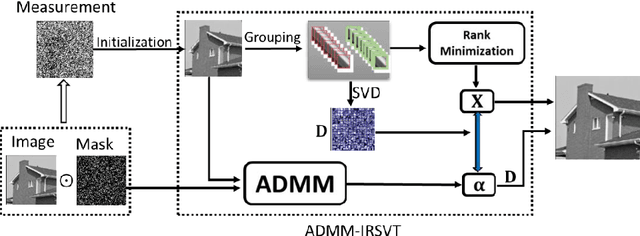
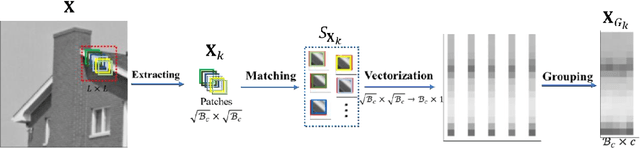


Abstract:Recently, low-rank matrix recovery theory has been emerging as a significant progress for various image processing problems. Meanwhile, the group sparse coding (GSC) theory has led to great successes in image restoration with group contains low-rank property. In this paper, we introduce a novel GSC framework using generalized rank minimization for image restoration tasks via an effective adaptive dictionary learning scheme. For a more accurate approximation of the rank of group matrix, we proposed a generalized rank minimization model with a generalized and flexible weighted scheme and the generalized nonconvex nonsmooth relaxation function. Then an efficient generalized iteratively reweighted singular-value function thresholding (GIR-SFT) algorithm is proposed to handle the resulting minimization problem of GSC. Our proposed model is connected to image restoration (IR) problems via an alternating direction method of multipliers (ADMM) strategy. Extensive experiments on typical IR problems of image compressive sensing (CS) reconstruction, inpainting, deblurring and impulsive noise removal demonstrate that our proposed GSC framework can enhance the image restoration quality compared with many state-of-the-art methods.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge