A Logical Semantics for PDDL+

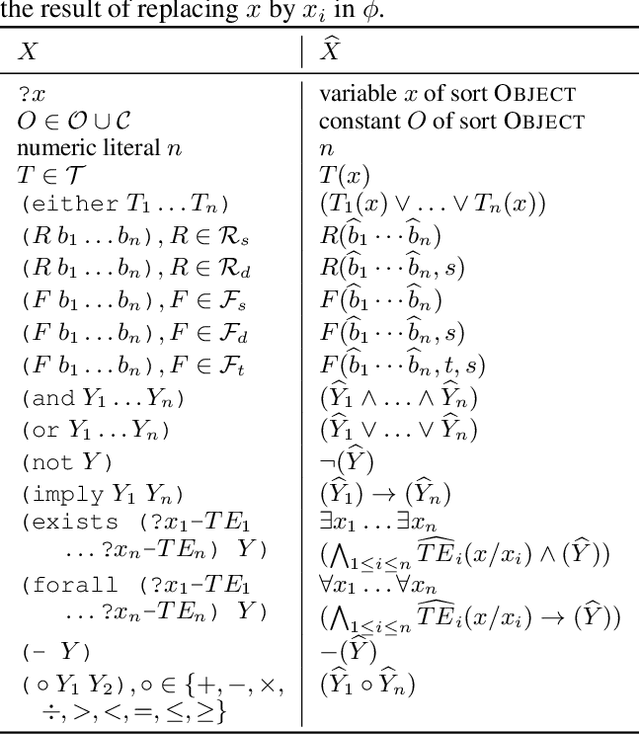
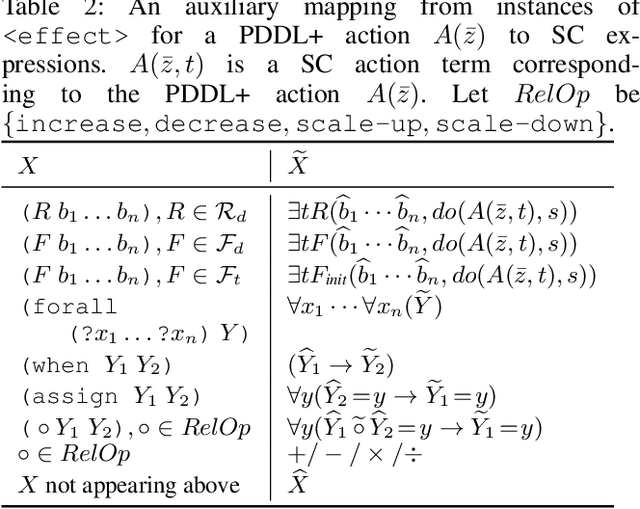
PDDL+ is an extension of PDDL2.1 which incorporates fully-featured autonomous processes and allows for better modelling of mixed discrete-continuous domains. Unlike PDDL2.1, PDDL+ lacks a logical semantics, relying instead on state-transitional semantics enriched with hybrid automata semantics for the continuous states. This complex semantics makes analysis and comparisons to other action formalisms difficult. In this paper, we propose a natural extension of Reiter's situation calculus theories inspired by hybrid automata. The kinship between PDDL+ and hybrid automata allows us to develop a direct mapping between PDDL+ and situation calculus, thereby supplying PDDL+ with a logical semantics and the situation calculus with a modern way of representing autonomous processes. We outline the potential benefits of the mapping by suggesting a new approach to effective planning in PDDL+.

Hybrid Temporal Situation Calculus

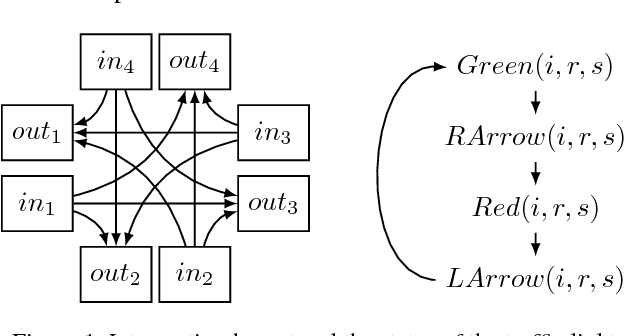
The ability to model continuous change in Reiter's temporal situation calculus action theories has attracted a lot of interest. In this paper, we propose a new development of his approach, which is directly inspired by hybrid systems in control theory. Specifically, while keeping the foundations of Reiter's axiomatization, we propose an elegant extension of his approach by adding a time argument to all fluents that represent continuous change. Thereby, we insure that change can happen not only because of actions, but also due to the passage of time. We present a systematic methodology to derive, from simple premises, a new group of axioms which specify how continuous fluents change over time within a situation. We study regression for our new temporal basic action theories and demonstrate what reasoning problems can be solved. Finally, we formally show that our temporal basic action theories indeed capture hybrid automata.