Translation of Algorithmic Descriptions of Discrete Functions to SAT with Applications to Cryptanalysis Problems

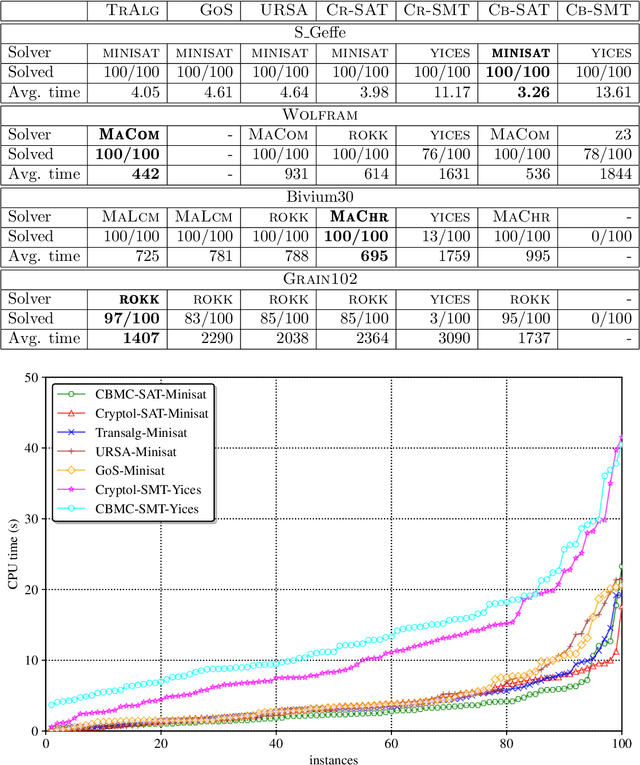
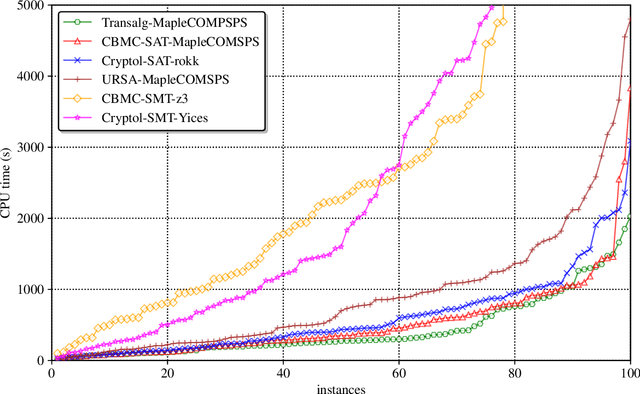
In the present paper we describe the technology for translating algorithmic descriptions of discrete functions to SAT. The proposed methods and algorithms of translation are aimed at application to the problems of SAT-based cryptanalysis. In the theoretical part of the paper we justify the main principles of general reduction to SAT for discrete functions from a class containing the majority of functions employed in cryptography. Based on these principles we describe the Transalg software system, developed with SAT-based cryptanalysis specifics in mind. We show the results of applications of Transalg to construction of a number of attacks on various cryptographic functions. Some of the corresponding attacks are state of the art. In the paper we also present the vast experimental data, obtained using the SAT-solvers that took first places at the SAT-competitions in the recent several years.

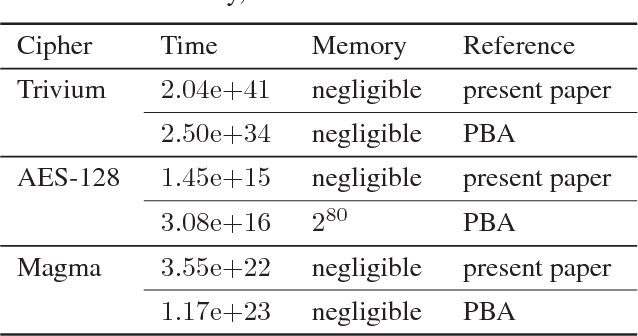
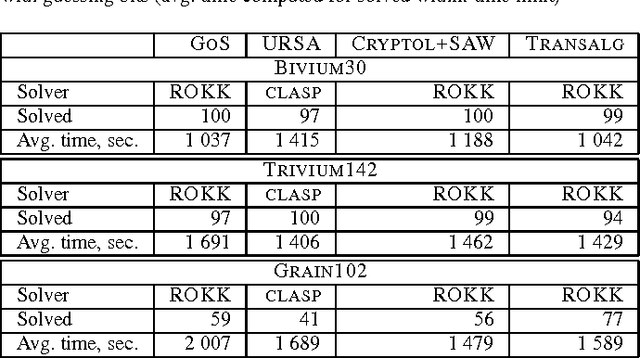
On Cryptographic Attacks Using Backdoors for SAT

Propositional satisfiability (SAT) is at the nucleus of state-of-the-art approaches to a variety of computationally hard problems, one of which is cryptanalysis. Moreover, a number of practical applications of SAT can only be tackled efficiently by identifying and exploiting a subset of formula's variables called backdoor set (or simply backdoors). This paper proposes a new class of backdoor sets for SAT used in the context of cryptographic attacks, namely guess-and-determine attacks. The idea is to identify the best set of backdoor variables subject to a statistically estimated hardness of the guess-and-determine attack using a SAT solver. Experimental results on weakened variants of the renowned encryption algorithms exhibit advantage of the proposed approach compared to the state of the art in terms of the estimated hardness of the resulting guess-and-determine attacks.

Encoding Cryptographic Functions to SAT Using Transalg System

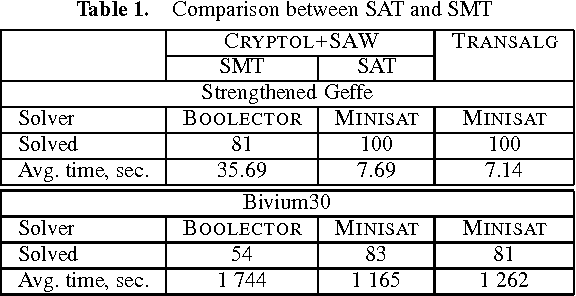
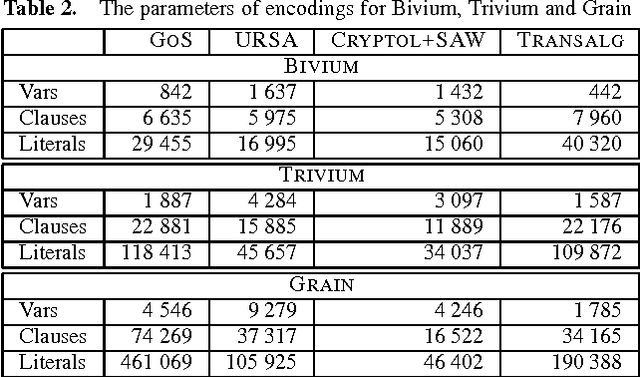
In this paper we propose the technology for constructing propositional encodings of discrete functions. It is aimed at solving inversion problems of considered functions using state-of-the-art SAT solvers. We implemented this technology in the form of the software system called Transalg, and used it to construct SAT encodings for a number of cryptanalysis problems. By applying SAT solvers to these encodings we managed to invert several cryptographic functions. In particular, we used the SAT encodings produced by Transalg to construct the family of two-block MD5 collisions in which the first 10 bytes are zeros. Also we used Transalg encoding for the widely known A5/1 keystream generator to solve several dozen of its cryptanalysis instances in a distributed computing environment. In the paper we compare in detail the functionality of Transalg with that of similar software systems.

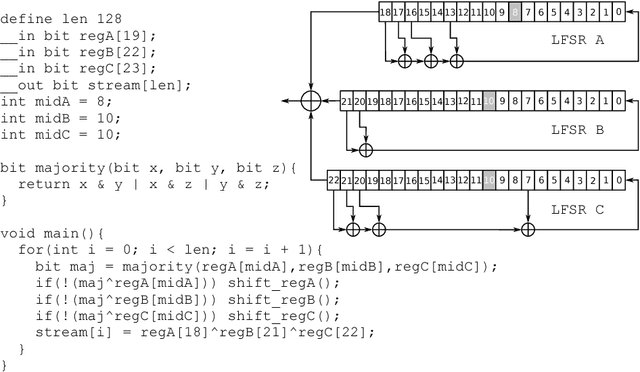
Transalg: a Tool for Translating Procedural Descriptions of Discrete Functions to SAT

In this paper we present the Transalg system, designed to produce SAT encodings for discrete functions, written as programs in a specific language. Translation of such programs to SAT is based on propositional encoding methods for formal computing models and on the concept of symbolic execution. We used the Transalg system to make SAT encodings for a number of cryptographic functions.

Using synchronous Boolean networks to model several phenomena of collective behavior

In this paper, we propose an approach for modeling and analysis of a number of phenomena of collective behavior. By collectives we mean multi-agent systems that transition from one state to another at discrete moments of time. The behavior of a member of a collective (agent) is called conforming if the opinion of this agent at current time moment conforms to the opinion of some other agents at the previous time moment. We presume that at each moment of time every agent makes a decision by choosing from the set {0,1} (where 1-decision corresponds to action and 0-decision corresponds to inaction). In our approach we model collective behavior with synchronous Boolean networks. We presume that in a network there can be agents that act at every moment of time. Such agents are called instigators. Also there can be agents that never act. Such agents are called loyalists. Agents that are neither instigators nor loyalists are called simple agents. We study two combinatorial problems. The first problem is to find a disposition of instigators that in several time moments transforms a network from a state where a majority of simple agents are inactive to a state with a majority of active agents. The second problem is to find a disposition of loyalists that returns the network to a state with a majority of inactive agents. Similar problems are studied for networks in which simple agents demonstrate the contrary to conforming behavior that we call anticonforming. We obtained several theoretical results regarding the behavior of collectives of agents with conforming or anticonforming behavior. In computational experiments we solved the described problems for randomly generated networks with several hundred vertices. We reduced corresponding combinatorial problems to the Boolean satisfiability problem (SAT) and used modern SAT solvers to solve the instances obtained.