Adynamical systems view of training generativemodels and the memorization phenomenon

Using recent works of one of the authors (VSB) on collapse in generative models and two time scale dynamics in stochastic gradient descent in high dimensions, we give a system theoretic explanation of the memorization phenomenon in generative models. This relies purely on the dynamic aspects of the training phase. Specifically, we use a result of Austin [2016] to motivate a stylized model for the loss function for stochastic gradient descent (SGD) wherein the loss function has a strong dependence on some variables and weak dependence on the rest in a precise sense. This naturally leads to two distinct time scales in the constant step size SGD that is commonly used in machine learning. This fact has been used to explain the double descent phenomenon in SGD in Borkar [2026]. In conjunction with a mathematical model for collapse phenomenon in SGD developed in Borkar [2025a], we analyze the constant step size SGD using the recent results of Azizian et al. [2024] in order to explain the phenomenon of memorization wherein a generative model that is concurrently being tuned yields the same or similar outputs for significant stretches of time. This gives a novel perspective on the aforementioned phenomena reported in machine learning literature and their interrelationships, using a dynamical systems viewpoint.

Path convergence of Markov chains on large graphs

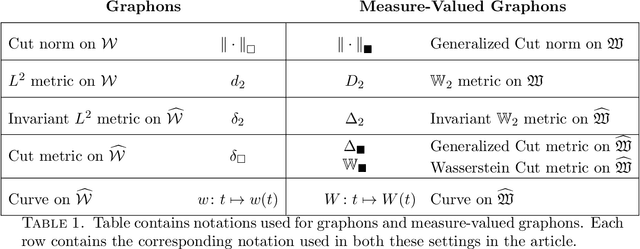
We consider two classes of natural stochastic processes on finite unlabeled graphs. These are Euclidean stochastic optimization algorithms on the adjacency matrix of weighted graphs and a modified version of the Metropolis MCMC algorithm on stochastic block models over unweighted graphs. In both cases we show that, as the size of the graph goes to infinity, the random trajectories of the stochastic processes converge to deterministic limits. These deterministic limits are curves on the space of measure-valued graphons. Measure-valued graphons, introduced by Lov\'{a}sz and Szegedy, are a refinement of the concept of graphons that can distinguish between two infinite exchangeable arrays that give rise to the same graphon limit. We introduce new metrics on this space which provide us with a natural notion of convergence for our limit theorems. This notion is equivalent to the convergence of infinite-exchangeable arrays. Under a suitable time-scaling, the Metropolis chain admits a diffusion limit as the number of vertices go to infinity. We then demonstrate that, in an appropriately formulated zero-noise limit, the stochastic process of adjacency matrices of this diffusion converge to a deterministic gradient flow curve on the space of graphons introduced in arXiv:2111.09459 [math.PR]. Under suitable assumptions, this allows us to estimate an exponential convergence rate for the Metropolis chain in a certain limiting regime. To the best of our knowledge, both the actual rate and the connection between a natural Metropolis chain commonly used in exponential random graph models and gradient flows on graphons are new in the literature.