Edge-Wise Graph-Instructed Neural Networks

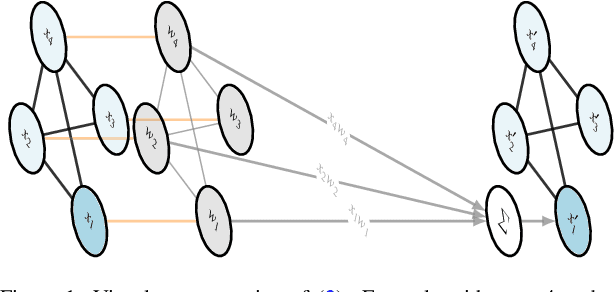
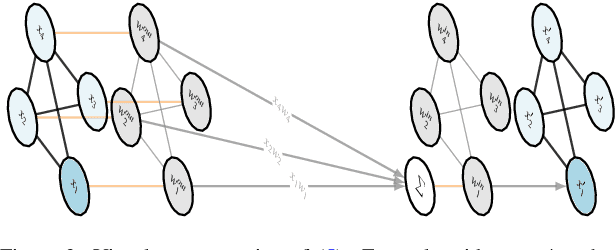
The problem of multi-task regression over graph nodes has been recently approached through Graph-Instructed Neural Network (GINN), which is a promising architecture belonging to the subset of message-passing graph neural networks. In this work, we discuss the limitations of the Graph-Instructed (GI) layer, and we formalize a novel edge-wise GI (EWGI) layer. We discuss the advantages of the EWGI layer and we provide numerical evidence that EWGINNs perform better than GINNs over graph-structured input data with chaotic connectivity, like the ones inferred from the Erdos-R\'enyi graph.

Graph-Informed Neural Networks for Sparse Grid-Based Discontinuity Detectors

In this paper, we present a novel approach for detecting the discontinuity interfaces of a discontinuous function. This approach leverages Graph-Informed Neural Networks (GINNs) and sparse grids to address discontinuity detection also in domains of dimension larger than 3. GINNs, trained to identify troubled points on sparse grids, exploit graph structures built on the grids to achieve efficient and accurate discontinuity detection performances. We also introduce a recursive algorithm for general sparse grid-based detectors, characterized by convergence properties and easy applicability. Numerical experiments on functions with dimensions n = 2 and n = 4 demonstrate the efficiency and robust generalization of GINNs in detecting discontinuity interfaces. Notably, the trained GINNs offer portability and versatility, allowing integration into various algorithms and sharing among users.