Mohsen Naghipourfar
Conditional out-of-sample generation for unpaired data using trVAE
Oct 30, 2019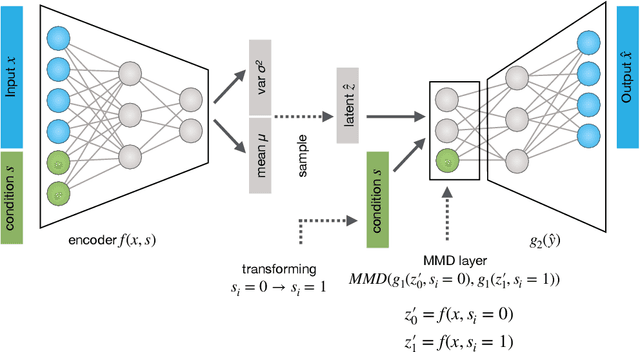
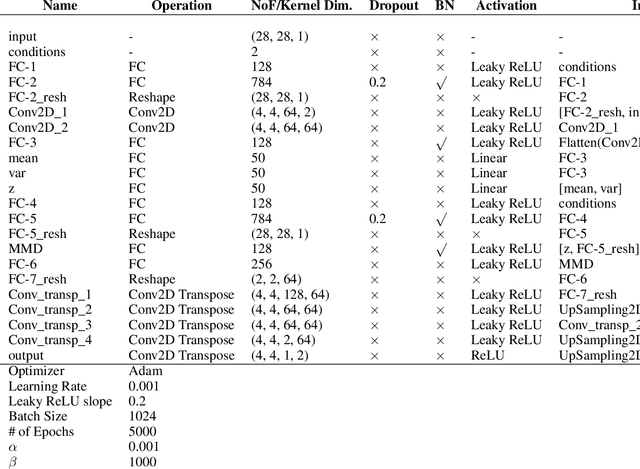
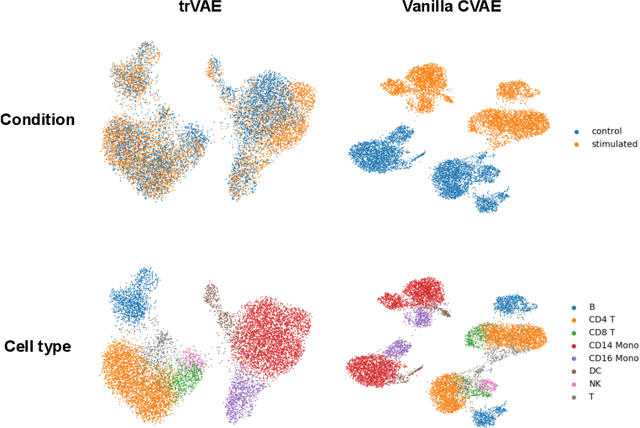
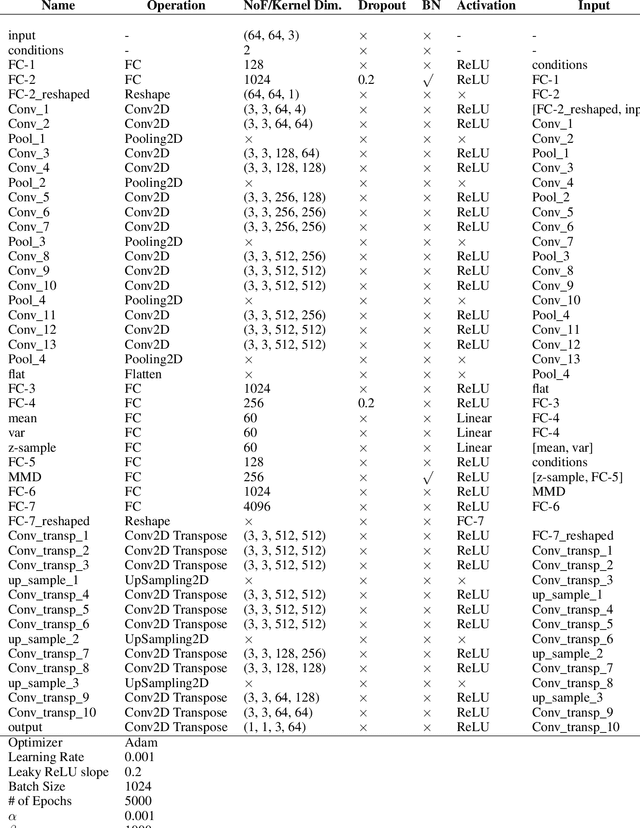
Abstract:While generative models have shown great success in generating high-dimensional samples conditional on low-dimensional descriptors (learning e.g. stroke thickness in MNIST, hair color in CelebA, or speaker identity in Wavenet), their generation out-of-sample poses fundamental problems. The conditional variational autoencoder (CVAE) as a simple conditional generative model does not explicitly relate conditions during training and, hence, has no incentive of learning a compact joint distribution across conditions. We overcome this limitation by matching their distributions using maximum mean discrepancy (MMD) in the decoder layer that follows the bottleneck. This introduces a strong regularization both for reconstructing samples within the same condition and for transforming samples across conditions, resulting in much improved generalization. We refer to the architecture as \emph{transformer} VAE (trVAE). Benchmarking trVAE on high-dimensional image and tabular data, we demonstrate higher robustness and higher accuracy than existing approaches. In particular, we show qualitatively improved predictions for cellular perturbation response to treatment and disease based on high-dimensional single-cell gene expression data, by tackling previously problematic minority classes and multiple conditions. For generic tasks, we improve Pearson correlations of high-dimensional estimated means and variances with their ground truths from 0.89 to 0.97 and 0.75 to 0.87, respectively.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge