Iterative Decoder of Channel-polarized Multilevel Coding for Data Center Networks

Data center networks (DCNs) require a low-cost, low-power optical transceiver to handle increased traffic from generative artificial intelligence, video streaming services, and more. Improving the required signal-to-noise ratio (RSNR) by digital signal processing such as forward error correction (FEC) mitigates the requirements for electrical and optical components. The optical transceivers in DCNs exploit a low-complexity soft-decision (SD) FEC, consisting of short block-length linear error-correcting codes and a low-complexity SD decoder (SDD), such as a Chase decoder and ordered statistical decoding. The low complexity SDD efficiently approaches a maximum likelihood decoding (MLD). However, the decoding performance of MLD is limited by its finite block length. In this paper, we describe the detail of our proposed channel-polarized multilevel coding with iterative decoding (CP-MLC-ID), which improves the decoding performance. The 19.5$\%$-OH CP-MLC-ID 128-bit extended Bose-Chaudhuri-Hocquenghem (eBCH) and KP4 codes outperform the concatenated eBCH and KP4 codes with a net coding gain of 0.25 and 0.40 dB for the same and double the number of SDDs, respectively. We also investigate the dependency of the decoding performance on the size of a bit interleaver. The performance degradation of CP-MLC-ID using an 8-bit interleaver is about 0.1 dB compared to using the large-bit interleaver. Our results indicate that even a weak connection by exclusive-OR between codewords improves the decoding performance, compared to simple concatenated codes in the DCNs.

Linear Least Squares Estimation of Fiber-Longitudinal Optical Power Profile

This paper presents a linear least squares method for fiber-longitudinal power profile estimation (PPE), which estimates an optical signal power distribution throughout a fiber-optic link at a coherent receiver. The method finds the global optimum in least square estimation of longitudinal power profiles, thus closely matching true optical power profiles and locating loss anomalies in a link with high spatial resolution. Experimental results show that the method achieves accurate PPE with an RMS error from OTDR of 0.18 dB. Consequently, it successfully identifies a loss anomaly as small as 0.77 dB, demonstrating the potential of a coherent receiver in locating even splice and connector losses. The method is also evaluated under a WDM condition with optimal system fiber launch power, highlighting its feasibility for use in operations. Furthermore, a fundamental limit for stable estimation and spatial resolution of least-squares-based PPE is quantitatively discussed in relation to the ill-posedness of PPE by evaluating the condition number of a nonlinear perturbation matrix.

Physics-oriented learning of nonlinear Schrödinger equation: optical fiber loss and dispersion profile identification

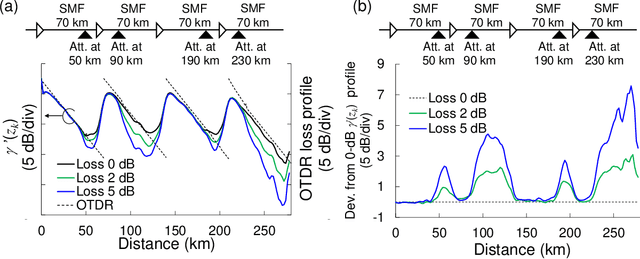
In optical fiber communication, system identification (SI) for the nonlinear Schr\"odinger equation (NLSE) has long been studied mainly for fiber nonlinearity compensation (NLC). One recent line of inquiry to combine a behavioral-model approach like digital backpropagation (DBP) and a data-driven approach like neural network (NN). These works are aimed for more NLC gain; however, by directing our attention to the learned parameters in such a SI process, system status information, i.e., optical fiber parameters, will possibly be extracted. Here, we show that the model-based optimization and interpretable nature of the learned parameters in NN-based DBP enable transmission line monitoring, fully extracting the actual in-line NLSE parameter distributions. Specifically, we demonstrate that longitudinal loss and dispersion profiles along a multi-span link can be obtained at once, directly from data-carrying signals without any dedicated analog devices such as optical time-domain reflectometry. We apply the method to a long-haul (~2,080 km) link and various link conditions are tested, including excess loss inserted, different fiber input power, and non-uniform level diagram. The measurement performance is also investigated in terms of measurement range, accuracy, and fiber launch power. These results provide a path toward simplified and automated network management as another application of DBP.