On Outer Bi-Lipschitz Extensions of Linear Johnson-Lindenstrauss Embeddings of Low-Dimensional Submanifolds of $\mathbb{R}^N$

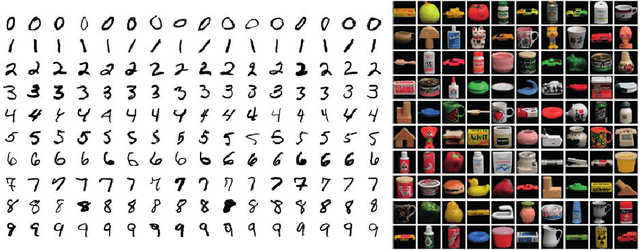
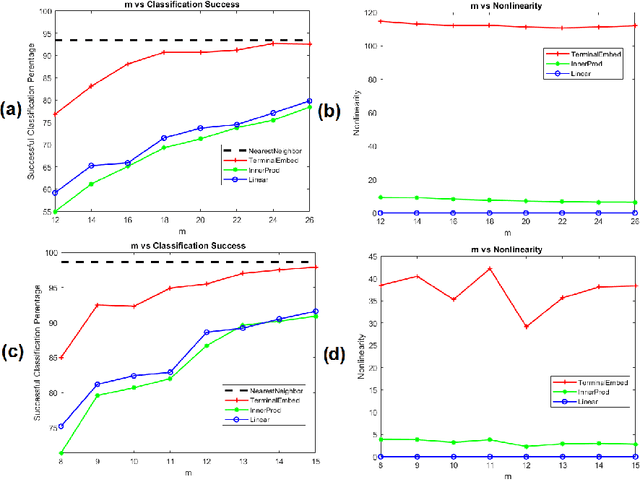
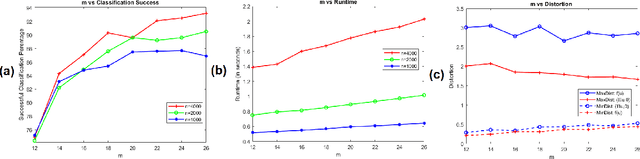
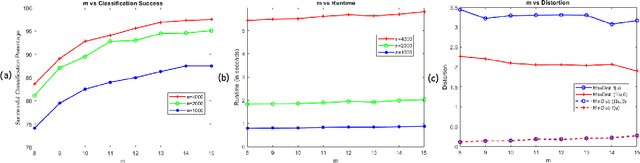
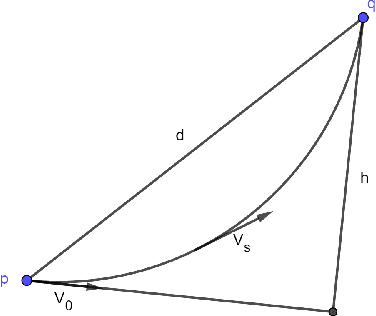
Let $\mathcal{M}$ be a compact $d$-dimensional submanifold of $\mathbb{R}^N$ with reach $\tau$ and volume $V_{\mathcal M}$. Fix $\epsilon \in (0,1)$. In this paper we prove that a nonlinear function $f: \mathbb{R}^N \rightarrow \mathbb{R}^{m}$ exists with $m \leq C \left(d / \epsilon^2 \right) \log \left(\frac{\sqrt[d]{V_{\mathcal M}}}{\tau} \right)$ such that $$(1 - \epsilon) \| {\bf x} - {\bf y} \|_2 \leq \left\| f({\bf x}) - f({\bf y}) \right\|_2 \leq (1 + \epsilon) \| {\bf x} - {\bf y} \|_2$$ holds for all ${\bf x} \in \mathcal{M}$ and ${\bf y} \in \mathbb{R}^N$. In effect, $f$ not only serves as a bi-Lipschitz function from $\mathcal{M}$ into $\mathbb{R}^{m}$ with bi-Lipschitz constants close to one, but also approximately preserves all distances from points not in $\mathcal{M}$ to all points in $\mathcal{M}$ in its image. Furthermore, the proof is constructive and yields an algorithm which works well in practice. In particular, it is empirically demonstrated herein that such nonlinear functions allow for more accurate compressive nearest neighbor classification than standard linear Johnson-Lindenstrauss embeddings do in practice.

On Fast Johnson-Lindenstrauss Embeddings of Compact Submanifolds of $\mathbb{R}^N$ with Boundary

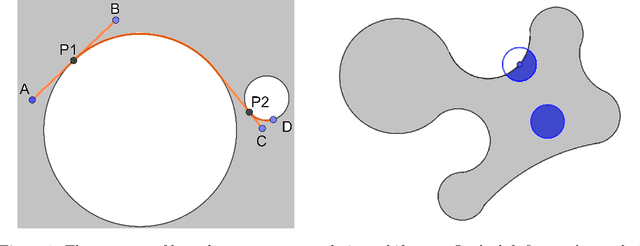
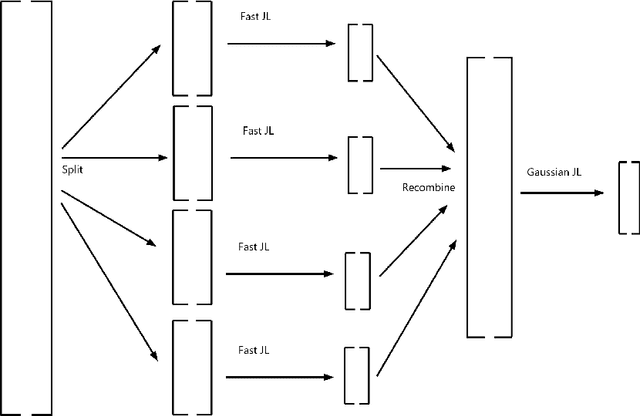
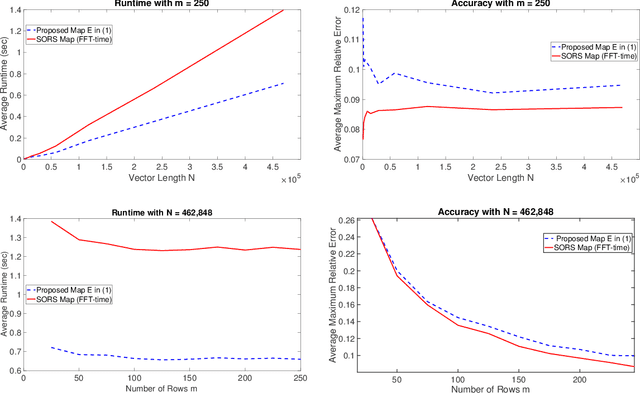
Let $\mathcal{M}$ be a smooth $d$-dimensional submanifold of $\mathbb{R}^N$ with boundary that's equipped with the Euclidean (chordal) metric, and choose $m \leq N$. In this paper we consider the probability that a random matrix $A \in \mathbb{R}^{m \times N}$ will serve as a bi-Lipschitz function $A: \mathcal{M} \rightarrow \mathbb{R}^m$ with bi-Lipschitz constants close to one for three different types of distributions on the $m \times N$ matrices $A$, including two whose realizations are guaranteed to have fast matrix-vector multiplies. In doing so we generalize prior randomized metric space embedding results of this type for submanifolds of $\mathbb{R}^N$ by allowing for the presence of boundary while also retaining, and in some cases improving, prior lower bounds on the achievable embedding dimensions $m$ for which one can expect small distortion with high probability. In particular, motivated by recent modewise embedding constructions for tensor data, herein we present a new class of highly structured distributions on matrices which outperform prior structured matrix distributions for embedding sufficiently low-dimensional submanifolds of $\mathbb{R}^N$ (with $d \lesssim \sqrt{N}$) with respect to both achievable embedding dimension, and computationally efficient realizations. As a consequence we are able to present, for example, a general new class of Johnson-Lindenstrauss embedding matrices for $\mathcal{O}(\log^c N)$-dimensional submanifolds of $\mathbb{R}^N$ which enjoy $\mathcal{O}(N \log (\log N))$-time matrix vector multiplications.