A Dynamical Estimation and Prediction for Covid19 on Romania using ensemble neural networks

In this paper, we propose an analysis of Covid19 evolution and prediction on Romania combined with the mathematical model of SIRD, an extension of the classical model SIR, which includes the deceased as a separate category. The reason is that, because we can not fully trust the reported numbers of infected or recovered people, we base our analysis on the more reliable number of deceased people. In addition, one of the parameters of our model includes the proportion of infected and tested versus infected. Since there are many factors which have an impact on the evolution of the pandemic, we decide to treat the estimation and the prediction based on the previous 7 days of data, particularly important here being the number of deceased. We perform the estimation and prediction using neural networks in two steps. Firstly, by simulating data with our model, we train several neural networks which learn the parameters of the model. Secondly, we use an ensemble of ten of these neural networks to forecast the parameters from the real data of Covid19 in Romania. Many of these results are backed up by a theorem which guarantees that we can recover the parameters from the reported data.

A regime switching on Covid19 analysis and prediction in Romania

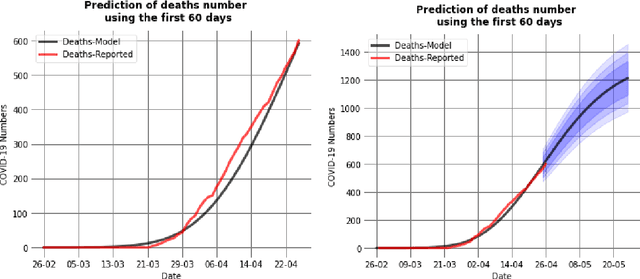
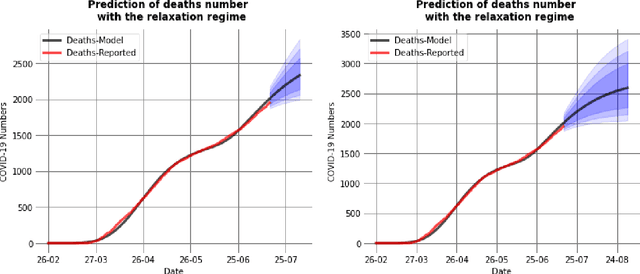
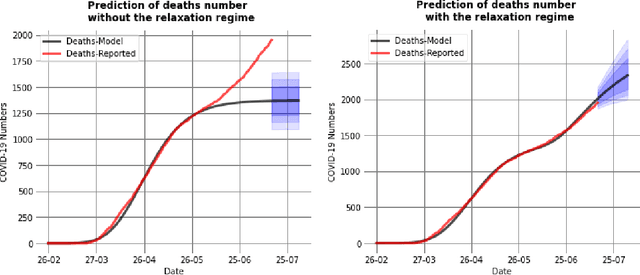
In this paper we propose a regime separation for the analysis of Covid19 on Romania combined with mathematical models of SIR and SIRD. The main regimes we study are, the free spread of the virus, the quarantine and partial relaxation and the last one is the relaxation regime. The main model we use is SIR which is a classical model, but because we can not fully trust the numbers of infected or recovered we base our analysis on the number of deceased people which is more reliable. To actually deal with this we introduce a simple modification of the SIR model to account for the deceased separately. This in turn will be our base for fitting the parameters. The estimation of the parameters is done in two steps. The first one consists in training a neural network based on SIR models to detect the regime changes. Once this is done we fit the main parameters of the SIRD model using a grid search. At the end, we make some predictions on what the evolution will be in a timeframe of a month with the fitted parameters.