Assessment of Data Consistency through Cascades of Independently Recurrent Inference Machines for fast and robust accelerated MRI reconstruction

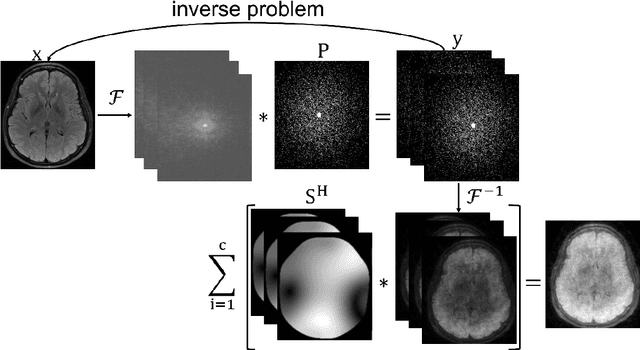
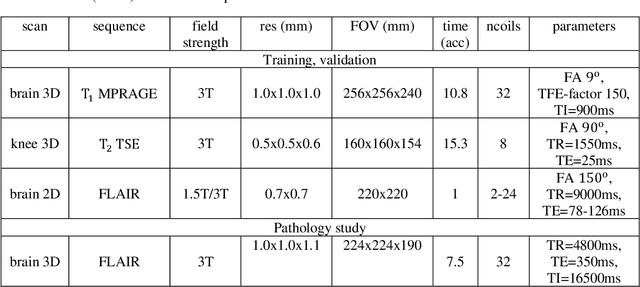
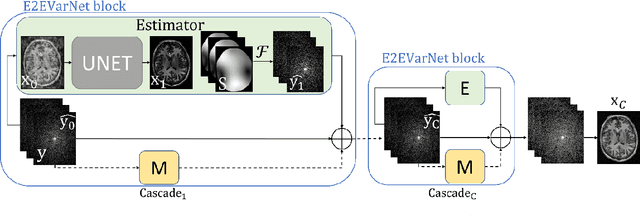
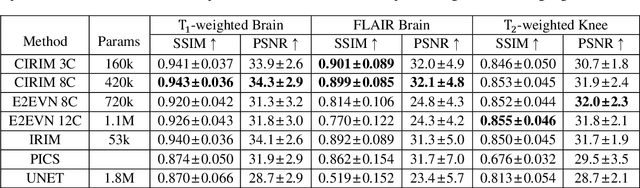
Interpretability and robustness are imperative for integrating Machine Learning methods for accelerated Magnetic Resonance Imaging (MRI) reconstruction in clinical applications. Doing so would allow fast high-quality imaging of anatomy and pathology. Data Consistency (DC) is crucial for generalization in multi-modal data and robustness in detecting pathology. This work proposes the Cascades of Independently Recurrent Inference Machines (CIRIM) to assess DC through unrolled optimization, implicitly by gradient descent and explicitly by a designed term. We perform extensive comparison of the CIRIM to other unrolled optimization methods, being the End-to-End Variational Network (E2EVN) and the RIM, and to the UNet and Compressed Sensing (CS). Evaluation is done in two stages. Firstly, learning on multiple trained MRI modalities is assessed, i.e., brain data with ${T_1}$-weighting and FLAIR contrast, and ${T_2}$-weighted knee data. Secondly, robustness is tested on reconstructing pathology through white matter lesions in 3D FLAIR MRI data of relapsing remitting Multiple Sclerosis (MS) patients. Results show that the CIRIM performs best when implicitly enforcing DC, while the E2EVN requires explicitly formulated DC. The CIRIM shows the highest lesion contrast resolution in reconstructing the clinical MS data. Performance improves by approximately 11% compared to CS, while the reconstruction time is twenty times reduced.

Recurrent Inference Machines as inverse problem solvers for MR relaxometry

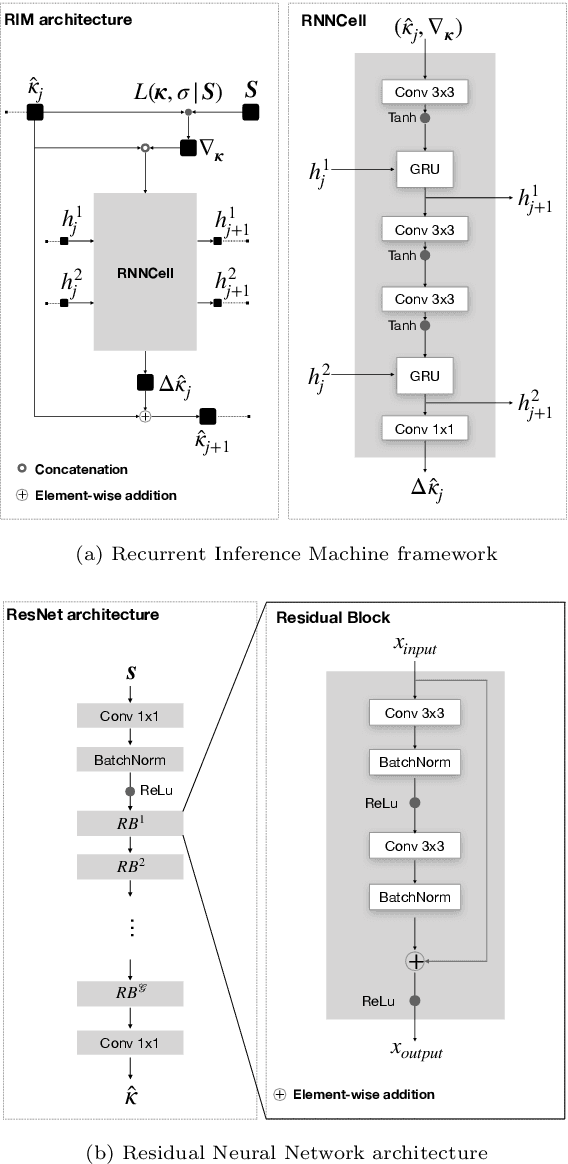
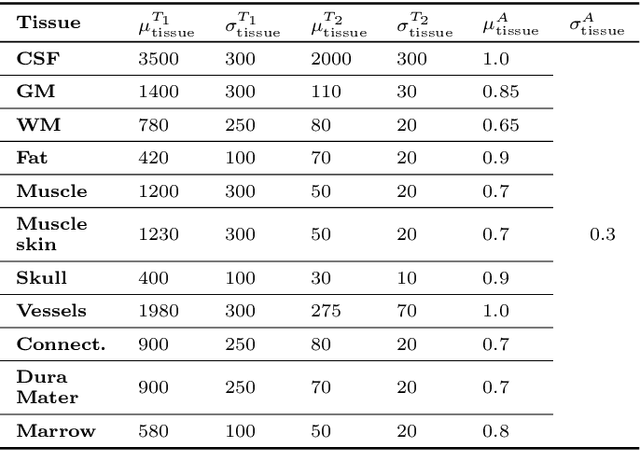
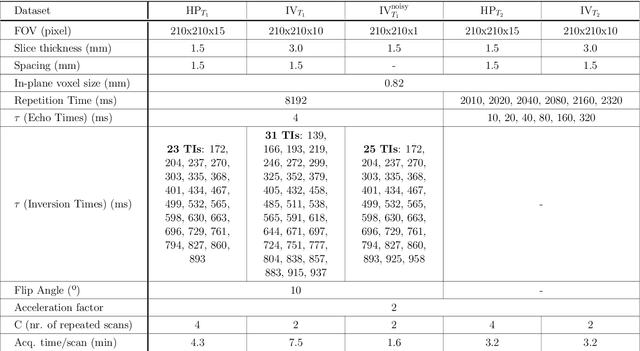
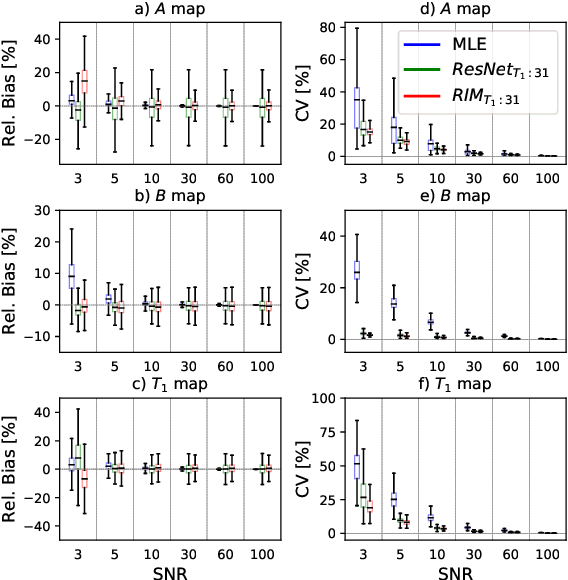
In this paper, we propose the use of Recurrent Inference Machines (RIMs) to perform T1 and T2 mapping. The RIM is a neural network framework that learns an iterative inference process based on the signal model, similar to conventional statistical methods for quantitative MRI (QMRI), such as the Maximum Likelihood Estimator (MLE). This framework combines the advantages of both data-driven and model-based methods, and, we hypothesize, is a promising tool for QMRI. Previously, RIMs were used to solve linear inverse reconstruction problems. Here, we show that they can also be used to optimize non-linear problems and estimate relaxometry maps with high precision and accuracy. The developed RIM framework is evaluated in terms of accuracy and precision and compared to an MLE method and an implementation of the ResNet. The results show that the RIM improves the quality of estimates compared to the other techniques in Monte Carlo experiments with simulated data, test-retest analysis of a system phantom, and in-vivo scans. Additionally, inference with the RIM is 150 times faster than the MLE, and robustness to (slight) variations of scanning parameters is demonstrated. Hence, the RIM is a promising and flexible method for QMRI. Coupled with an open-source training data generation tool, it presents a compelling alternative to previous methods.