Intelligent Meta-Imagers: From Compressed to Learned Sensing

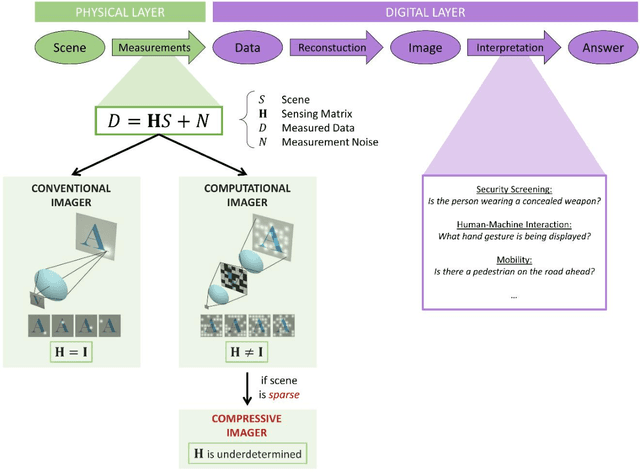
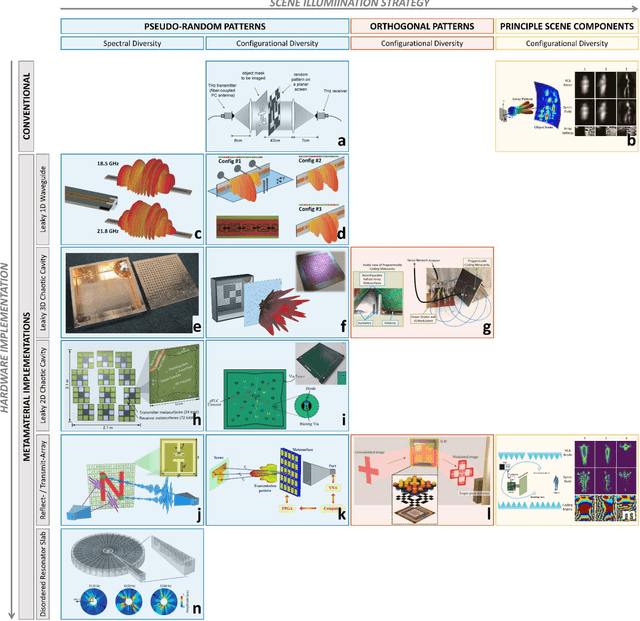
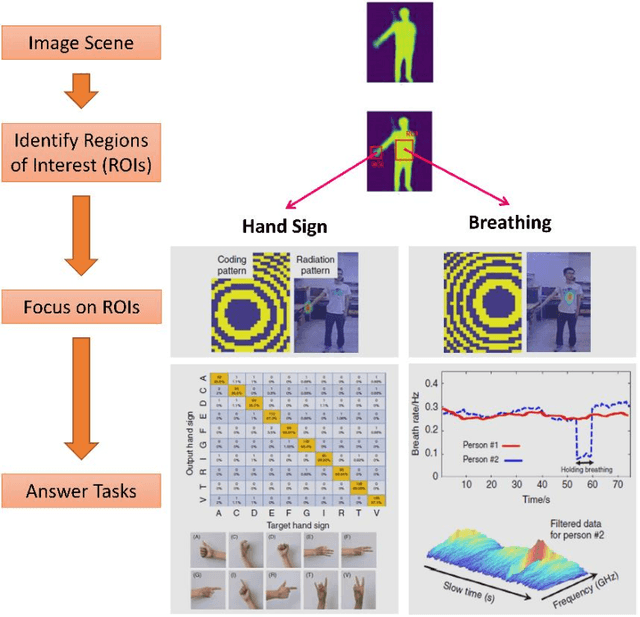
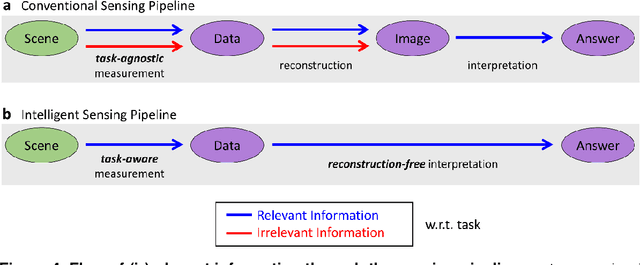
Computational meta-imagers synergize metamaterial hardware with advanced signal processing approaches such as compressed sensing. Recent advances in artificial intelligence (AI) are gradually reshaping the landscape of meta-imaging. Most recent works use AI for data analysis, but some also use it to program the physical meta-hardware. The role of "intelligence" in the measurement process and its implications for critical metrics like latency are often not immediately clear. Here, we comprehensively review the evolution of computational meta-imaging from the earliest frequency-diverse compressive systems to modern programmable intelligent meta-imagers. We introduce a clear taxonomy in terms of the flow of task-relevant information that has direct links to information theory: compressive meta-imagers indiscriminately acquire all scene information in a task-agnostic measurement process that aims at a near-isometric embedding; intelligent meta-imagers highlight task-relevant information in a task-aware measurement process that is purposefully non-isometric. We provide explicit design tutorials for the integration of programmable meta-atoms as trainable physical weights into an intelligent end-to-end sensing pipeline. This merging of the physical world of metamaterial engineering and the digital world of AI enables the remarkable latency gains of intelligent meta-imagers. We further outline emerging opportunities for cognitive meta-imagers with reverberation-enhanced resolution and we point out how the meta-imaging community can reap recent advances in the vibrant field of metamaterial wave processors to reach the holy grail of low-energy ultra-fast all-analog intelligent meta-sensors.

Performance Analysis and Dynamic Evolution of Deep Convolutional Neural Network for Nonlinear Inverse Scattering

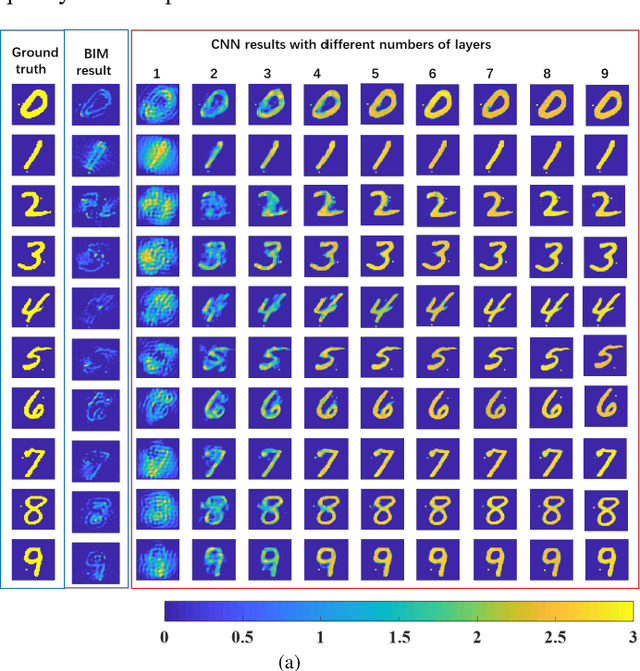
The solution of nonlinear electromagnetic (EM) inverse scattering problems is typically hindered by several challenges such as ill-posedness, strong nonlinearity, and high computational costs. Recently, deep learning has been demonstrated to be a promising tool in addressing these challenges. In particular, it is possible to establish a connection between a deep convolutional neural network (CNN) and iterative solution methods of nonlinear EM inverse scattering. This has led to the development of an efficient CNN-based solution to nonlinear EM inverse problems, termed DeepNIS. It has been shown that DeepNIS can outperform conventional nonlinear inverse scattering methods in terms of both image quality and computational time. In this work, we quantitatively evaluate the performance of DeepNIS as a function of the number of layers using structure similarity measure (SSIM) and mean-square error (MSE) metrics. In addition, we probe the dynamic evolution behavior of DeepNIS by examining its near-isometry property. It is shown that after a proper training stage the proposed CNN is near optimal in terms of the stability and generalization ability.

Fast Algorithm of High-resolution Microwave Imaging Using the Non-parametric Generalized Reflectivity Model

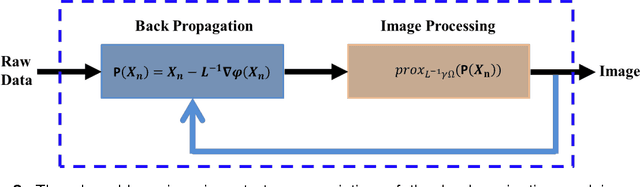
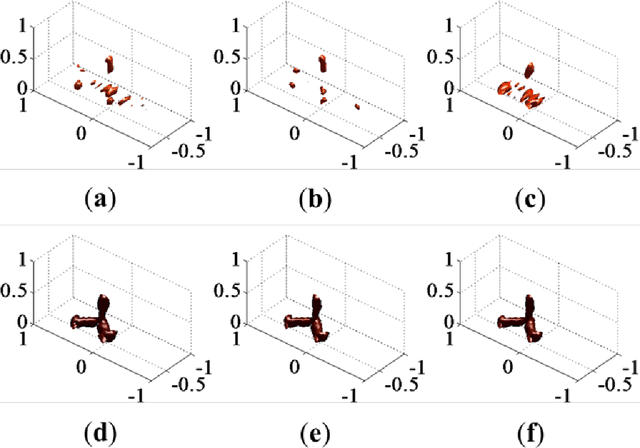
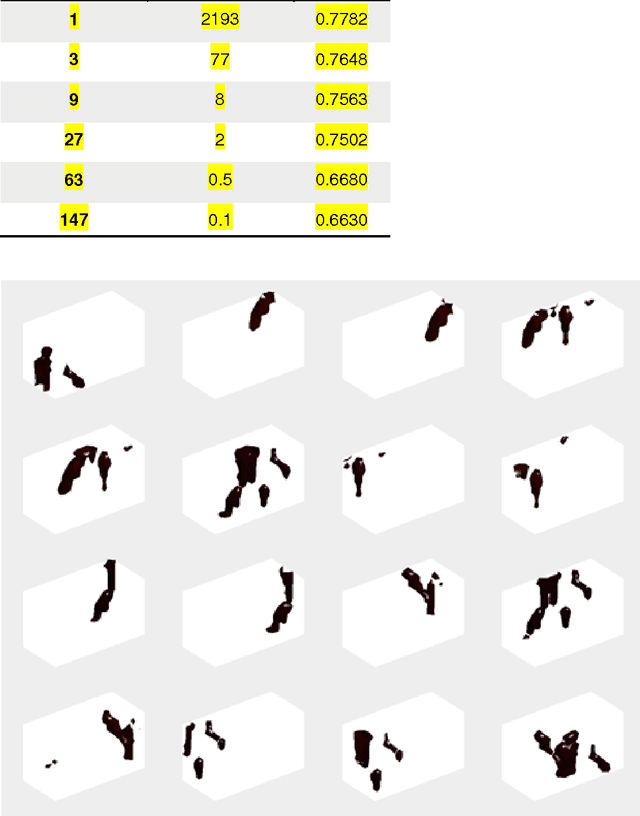
This paper presents an efficient algorithm of high-resolution microwave imaging based on the concept of generalized reflectivity. The contribution made in this paper is two-fold. We introduce the concept of non-parametric generalized reflectivity (GR, for short) as a function of operational frequencies and view angles, etc. The GR extends the conventional Born-based imaging model, i.e., single-scattering model, into that accounting for more realistic interaction between the electromagnetic wavefield and imaged scene. Afterwards, the GR-based microwave imaging is formulated in the convex of sparsity-regularized optimization. Typically, the sparsity-regularized optimization requires the implementation of iterative strategy, which is computationally expensive, especially for large-scale problems. To break this bottleneck, we convert the imaging problem into the problem of physics-driven image processing by introducing a dual transformation. Moreover, this image processing is performed over overlapping patches, which can be efficiently solved in the parallel or distributed manner. In this way, the proposed high-resolution imaging methodology could be applicable to large-scale microwave imaging problems. Selected simulation results are provided to demonstrate the state-of-art performance of proposed methodology.