Learning When to Sample: Confidence-Aware Self-Consistency for Efficient LLM Chain-of-Thought Reasoning

Large language models (LLMs) achieve strong reasoning performance through chain-of-thought (CoT) reasoning, yet often generate unnecessarily long reasoning paths that incur high inference cost. Recent self-consistency-based approaches further improve accuracy but require sampling and aggregating multiple reasoning trajectories, leading to substantial additional computational overhead. This paper introduces a confidence-aware decision framework that analyzes a single completed reasoning trajectory to adaptively select between single-path and multi-path reasoning. The framework is trained using sentence-level numeric and linguistic features extracted from intermediate reasoning states in the MedQA dataset and generalizes effectively to MathQA, MedMCQA, and MMLU without additional fine-tuning. Experimental results show that the proposed method maintains accuracy comparable to multi-path baselines while using up to 80\% fewer tokens. These findings demonstrate that reasoning trajectories contain rich signals for uncertainty estimation, enabling a simple, transferable mechanism to balance accuracy and efficiency in LLM reasoning.

Doubly-Valid/Doubly-Sharp Sensitivity Analysis for Causal Inference with Unmeasured Confounding

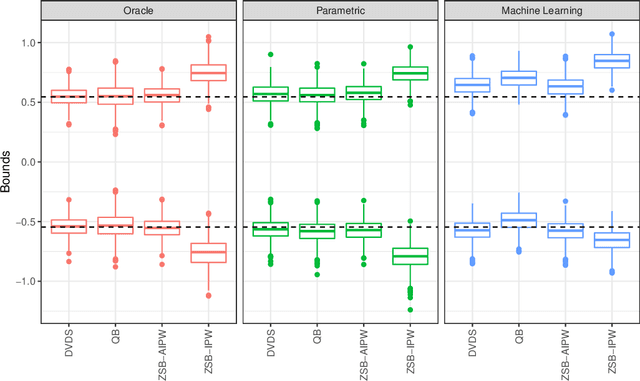
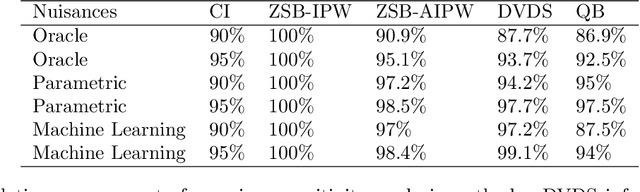
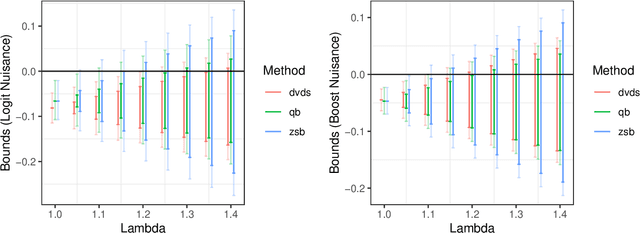
We study the problem of constructing bounds on the average treatment effect in the presence of unobserved confounding under the marginal sensitivity model of Tan (2006). Combining an existing characterization involving adversarial propensity scores with a new distributionally robust characterization of the problem, we propose novel estimators of these bounds that we call "doubly-valid/doubly-sharp" (DVDS) estimators. Double sharpness corresponds to the fact that DVDS estimators consistently estimate the tightest possible (i.e., sharp) bounds implied by the sensitivity model even when one of two nuisance parameters is misspecified and achieve semiparametric efficiency when all nuisance parameters are suitably consistent. Double validity is an entirely new property for partial identification: DVDS estimators still provide valid, though not sharp, bounds even when most nuisance parameters are misspecified. In fact, even in cases when DVDS point estimates fail to be asymptotically normal, standard Wald confidence intervals may remain valid. In the case of binary outcomes, the DVDS estimators are particularly convenient and possesses a closed-form expression in terms of the outcome regression and propensity score. We demonstrate the DVDS estimators in a simulation study as well as a case study of right heart catheterization.